Suppose (Y_{i}=beta_{0}+beta_{1} X_{i}+u_{i}), where (left(X_{i}, u_{i} ight)) are i.i.d. and (X_{i}) is a Bernoulli random variable with
Question:
Suppose \(Y_{i}=\beta_{0}+\beta_{1} X_{i}+u_{i}\), where \(\left(X_{i}, u_{i}\right)\) are i.i.d. and \(X_{i}\) is a Bernoulli random variable with \(\operatorname{Pr}(X=1)=0.20\). When \(X=1, u_{i}\) is \(N(0,4)\); when \(X=0, u_{i}\) is \(N(0,1)\).
a. Show that the regression assumptions in Key Concept 4.3 are satisfied.
b. Derive an expression for the large-sample variance of \(\hat{\beta}_{1}\). Evaluate the terms in Equation (4.19).
Equation (4.19)
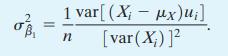
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





