Allow two-party competition in the elections over infinite time horizon, (t=0,1, ldots,+infty). Assume that there is an election every other period and two parties, (i=L,

Question

78 users unlocked this solution today!

Allow two-party competition in the elections over infinite time horizon, \(t=0,1, \ldots,+\infty\). Assume that there is an election every other period and two parties, \(i=L, R\) ("left" and "right"), compete in the election period to get into power.

Assume that in each period \(t\) the party in power has the opportunity to conduct a credit policy, providing an extra credit supply of \(L_{t}\) to the economy through banks, and assume that an extra \(1 \%\) increase in credit supply \(L_{t}\) leads to \(1 \%\) increase in GDP, \(Y_{t}\). We use lower case alphabets to denote logarithm forms of \(L_{t}\) and \(Y_{t}\), i.e.,

\[l_{t}=\ln L_{t} \text {, and } y_{t}=\ln Y_{t} \text {. }\]

In each period \(t\), voters have an expectation on total output, or, GDP, denoted by \(y_{\tau}^{e}\). With realized GDP \(y_{\tau}\), the surprise to the voters is denoted by

\[\delta_{t}=y_{t}-y_{t}^{e}=l_{t}-l_{t}^{e} .\]

Voters are more likely to vote for the ruling party, if they are positively surprised. On the other hand, a convex political cost is incurred for the credit policy, denoted by a cost function \(c\left(l_{t}\right)\)

\[c\left(l_{t}\right)=\frac{\theta}{2} l_{t}^{2}\]

with \(\theta\) being a constant and \(\theta>0\). To balance the gain and the cost, a ruling party \(i\) has targets on \(\delta\) and \(l\), call them \(\bar{\delta}^{i}\) and \(\bar{l}^{i}\), and the loss function for a ruling party in an election cycle is given by

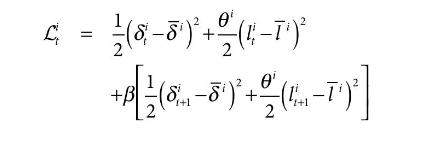with \(\theta^{i}\) being a constant for party \(i\) and \(\theta^{i}>0, \beta\) being a constant discount factor and \(0& \bar{\delta}^{L} \geq \bar{\delta}^{R} \\
& \bar{l}^{L} \geq \bar{l}^{R} \\
& \theta^{L} \leq \theta^{R} .
\end{aligned}\]
We further assume that voters are rational such that \[l_{t}^{e}=E_{t-1}\left[l_{t}\right] .\]

(a) Compute the optimal choice on \(l_{t}\) for each party, i.e., \(l_{t}^{L}\) and \(l_{t}^{R}\), respectively.

(b) Assume that the probability that party \(L\) wins in period \(t\) is exogenously given as \(p^{L}\). Then before the election, voters' expectation on credit supply can be also expressed as \[l_{t}^{e}=p^{L} l_{t}^{L}+\left(1-p^{L}\right) l_{t}^{R}\]
Compute \(l_{t}^{L}\) and \(l_{t}^{R}\) as functions of \(p^{L}\).

(c) What is the difference in economic performance, between party \(L\) 's and party \(R\) 's first period in power? Does the difference persist in their second period?

201 (8-5)+(-7) +8 (8-8) (LT)}] 1+1 1+1