Consider a simple life-cycle model, in which individuals live for two periods; they work in the first, saving for the second. Let C₁ denote consumption in the first period and C₂ in the second; r, the interest rate, and w, the wage. Then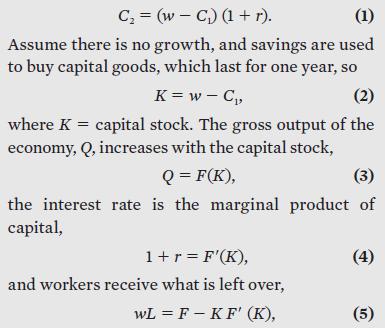

where L is the labor supply, which for convenience, we set equal to 1.

Individuals supply labor inelastically, but decide how much to consume by maximizing U(C₁, C₂), the two-period utility function, subject to equation 1. Describe the market equilibrium. You may fi nd the following graphical interpretation useful. Put C₂ on the vertical axis and C₁ on the horizontal. Plot the set of feasible combinations, using equations 1 through 5. For each K there is a value of the wage; for each value of consumption in the two periods there is a value of the marginal rate of substitution, the amount of consumption while working that individuals are willing to trade off for more consumption in retirement. In equilibrium, the marginal rate of substitution equals the interest rate. Through any point on the feasibility locus, you can draw a line with slope equal to 1 + r (this just depends on K), and you can draw the in difference curve. The equilibrium point along the feasibility locus is the point where the two are tangent at that point. Now, explore how a pay-as-you-go Social Security system changes the equilibrium. Let T be the transfer from the younger generation to the older. Now C₂ = (w - T - C₁)(1 + r) 1 T. Show how the equilibrium may be affected, and why the capital stock may be smaller.

Transcribed Image Text:

C₂ = (wC) (1 + r). (1) Assume there is no growth, and savings are used to buy capital goods, which last for one year, so K = w - C₂, (2) where K = capital stock. The gross output of the economy, Q, increases with the capital stock, Q = F(K), (3) the interest rate is the marginal product of capital, 1 + r = F'(K), and workers receive what is left over, wL = F - K F' (K), (4) (5)