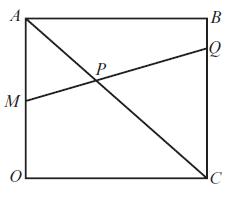
OABC is a square. M is the midpoint of OA, and Q divides BC in the ratio
Question:
OABC is a square. M is the midpoint of OA, and Q divides BC in the ratio 1 : 3. AC and MQ meet at P.
a. If OA(vector) = a and OC(vector) = c, express OP(vector) in terms of a and c.
b. Show that P divides AC in the ratio 2 : 3.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
To solve this problem lets break it down step by step a To find OPvector in terms of a and c we need to analyze the given information Since M is the m...View the full answer
Answered By
ROXAN VEGA
I have extensive hands-on experience and proficiency in assisting students in various subjects. During my tutoring sessions, I prioritize creating a supportive and engaging learning environment. I strive to understand each student's unique learning style and tailor my teaching methods accordingly. I am patient, empathetic, and skilled at breaking down complex concepts into simpler, more manageable parts. I encourage active participation and discussion, promoting critical thinking and problem-solving skills.
One of my strengths as a tutor is my ability to adapt to different learning needs and styles. I can explain difficult concepts using real-life examples, visual aids, or interactive exercises, depending on what works best for the student. I also provide clear explanations, answer questions, and offer guidance in a clear and concise manner.
0 Reviews
10+ Question Solved
Related Book For 
Edexcel AS And A Level Mathematics Pure Mathematics Year 1/AS
ISBN: 9781292183398
1st Edition
Authors: Greg Attwood
Question Posted:
