Starting with the Navier equations (10.2.2) for plane strain, introduce the complex displacement U = u +

Question:

Starting with the Navier equations (10.2.2) for plane strain, introduce the complex displacement U = u + iv, and show that:

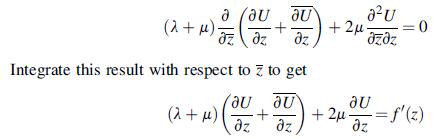
a (au au z (2 + ) = (12 + az dz Integrate this result with respect to Z to get au au z z (2+) + a U +2 zaz zz

where f^′(z) is an arbitrary analytic function of z. Next, combining both the preceding equation and its conjugate, solve for ∂U/∂z, and simplify to get form (10.2.9).

Equation 10.2.2

Equation 10.2.9

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For answer-question

Elasticity Theory Applications And Numerics

ISBN: 9780128159873

4th Edition

Authors: Martin H. Sadd Ph.D.

See More Books

Question Posted: Jan 15, 2024 05:01 AM

See More Questions

Managing Scope Changes Case Study Scope changes on a project can occur regardless of how well the project is planned or executed. Scope changes can be the result of something that was omitted during...
The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...
Consider the case of a crack problem in an anisotropic monoclinic material under anti-plane deformation as described in Exercise 11.16. Following relation (11.6.6), choose the complex potential form...
How does the trade-off between decision management and decision control affect the form that an absorption cost system takes within a particular firm?
At a certain instant, four particles have the xy coordinates and velocities given in the following table. At that instant, what are the (a) X and (b) Y coordinates of their center of mass and (c) The...
In a statement to Gillette's shareholders, its CEO indicated, "Despite several new product launches, Gillette's advertising-to-sales declined dramatically . . . to 7.5 percent last year. Gillette's...
Refer to Samsungs financial statements in Appendix A. Compute its debt ratio as of December 31, 2015, and December 31, 2014. Data From Samsung Financial Statement Appendix A Samsung Electronics Co.,...
A company has sales of $1,000,000, variable costs of $250,000, and fixed costs of $600,000. Compute the following: 1. Contribution margin ratio. 2. Break-even sales volume. 3. Margin of safety ratio....
What is operating leverage and how is it related to the cost structure of any organisation ?
Establish the relations: [2(u +iv)] = [2Y(3) + (3)] = (ex - e + 2iexy) where ex, ey, and exy are the strain components.
Formally integrate relation (10.2.6) and establish the result: Equation 10.2.6 (z, Z) = 1/2 (zy (z) + y (2) + V (2) + (2) Re(Zy(z)+(z))
1. The probability of getting three heads in five tosses of a coin is .3125. What are the odds of getting three heads? 2. The probability that a graduate of a Big Ten school will eventually earn a...
What five elements comprise the strategy formulation diamond?
A supply chain is a specific order of steps taken by a company to get its product or service to the public. Supply chain management involves managing the flows of goods and services by linking...
The Languedoc-Roussillon region in southern France is home to three vineyards. Chteau Beauregard Mirouze produces 2,500 bottles of wine per month at a total cost of 40,000. Chteau de Bruthel produces...
South African Airways (SAA) and Qantas Airlines held a duopoly on direct flights from Australia to South Africa. In 2014, Australias International Air Services Commission (IASC) indicated that the...
What are the internal and external perspectives of competitive advantage?
What are the differences between the gross, operating, and net profit margins?
6 (a) Briefly develop a mathematical model of the behaviour of a copper-twisted pair cable (b) Derive the magnetic energy from: w given that: K + w, where the - - k symbols have their usual meaning...
Beginning from their definitions, determine the conversion factor between ft lb and kW h in Table 7.1. Table 7.1 ft- Ib Btu kW -h 1 1.356 1.285 x 10-3 3.766 x 10-7 0.7376 1 9.478 x 10-4 2.778 x...
A remote-controlled toy car weighs 3 lb and moves at 15 ft/s. What is its kinetic energy?
A lawn mower engine is started by pulling a cord wrapped around a hub of radius 6.0 cm. If a constant tension of 80 N is maintained in the cord and the hub makes three revolutions before the motor...
Prepare compound journal entries for each transaction. a. The owner invests $10,000 cash and $7,000 of equipment in the company in exchange for common stock. b. The company acquires $5,500 of...
A company had the following assets and liabilities at the beginning and end of this year. Beginning of the year End of the year Assets $ 121,500 166,500 Liabilities $ 52,087 67,433 a. Owner made no...
According to the producer price index database maintained by the Bureau of Labor Statistics, the average cost of computer equipment fell 3.8 percent between January and December 2018. Let's see...

Starting with the Navier equations (10.2.2) for plane strain, introduce the complex displacement U = u +

Question:

a (au au z (2 + ) = (12 + az dz Integrate this result with respect to Z to get au au z z (2+) + a U +2 zaz zz au +2- = 0 -= f'(z) z

Step by Step Answer:

Use Navier Equations Vu2VVu0 to construct the complex form uPu 2 ...View the full answer

Elasticity Theory Applications And Numerics

Students also viewed these Engineering questions