Using integral tables verify that the gradient Flamant stress integral solution in Example 15.17 reduces to the classical elasticity form as per equations (15.7.20).

Data from example 15.17

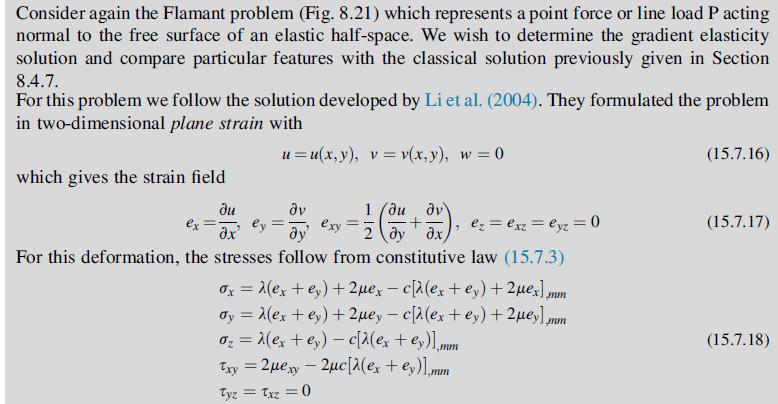
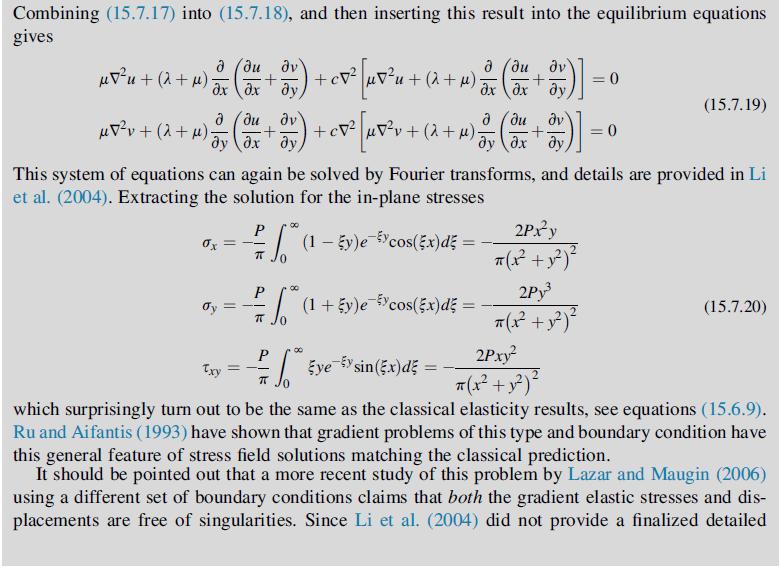
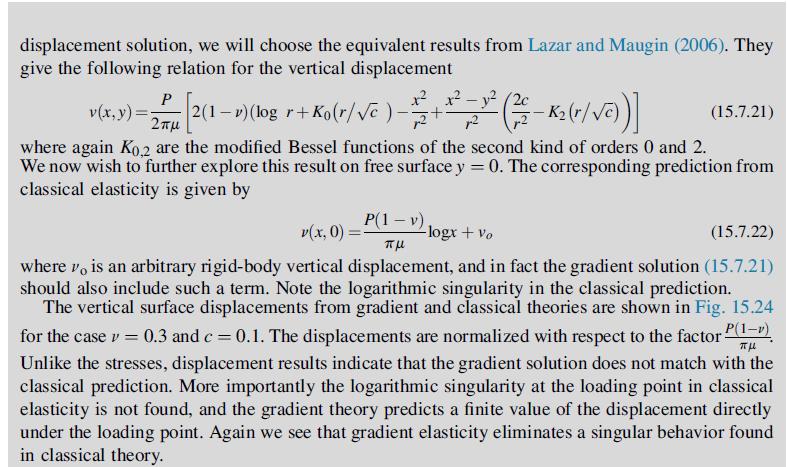
Equation 15.7.20

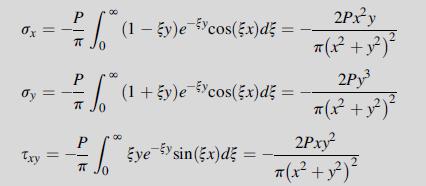
Transcribed Image Text:

Consider again the Flamant problem (Fig. 8.21) which represents a point force or line load P acting normal to the free surface of an elastic half-space. We wish to determine the gradient elasticity solution and compare particular features with the classical solution previously given in Section 8.4.7. For this problem we follow the solution developed by Li et al. (2004). They formulated the problem in two-dimensional plane strain with u= u(x,y), v=v(x,y), w=0 which gives the strain field v dy' For this deformation, the stresses follow from constitutive law (15.7.3) x' = 1/ -1/2 (3H + 3x), dy ax = exy e = exz = Cyz = 0 ox=2(ex + ey) +2ex - c[2(ex + ey) +2ex].mm oy = 2(ex + ey) +2ey - c[2(ex + ey) +2ey] mm o = 2(ex + ey) - c[2(ex + ey)],mm Txy = 2exy - 2uc [2(ex + ey)],mm Tyz = Txz = 0 (15.7.16) (15.7.17) (15.7.18)