For the domain sampling model described in Section10.4.1, show (a) If (operatorname{Var}left(y_{k} ight)=sigma^{2}) is a constant, the

For the domain sampling model described in Section10.4.1, show

(a) If \(\operatorname{Var}\left(y_{k}\right)=\sigma^{2}\) is a constant, the Spearman-Brown \(ho_{K}\) and Cronbach coefficient alpha \(\alpha_{K}\) are identical;

(b) If \(\operatorname{Cov}\left(y_{k}, y_{l}\right) \geq c>0\) for all \(1 \leq k, l \leq K\), then \(\lim _{K \rightarrow \infty} \alpha_{K}=1\);

(c) Choose a setting where \(\sigma_{k}^{2}=\operatorname{Var}\left(y_{k}\right)\) is a function of \(k\) and compare the estimates of \(ho_{K}\) and \(\alpha_{K}\) using Monte Carlo simulation with a sample size 5,000.

Section 10.4.1

Transcribed Image Text:

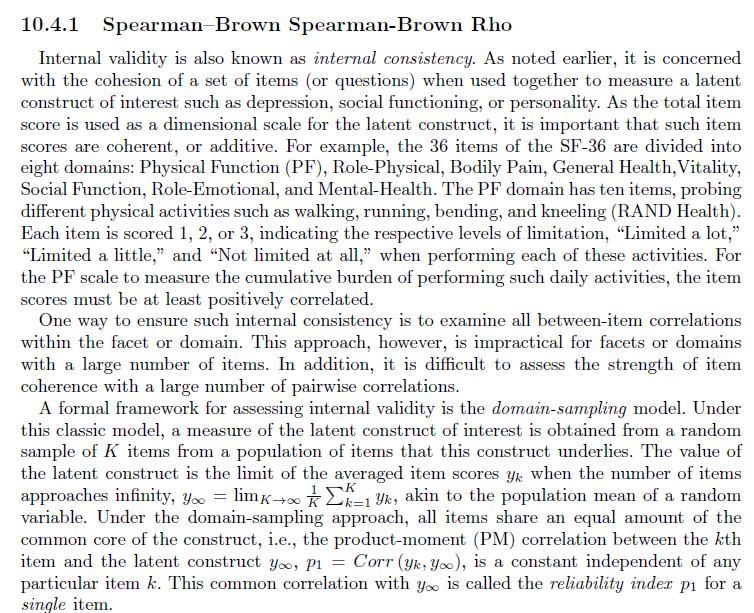
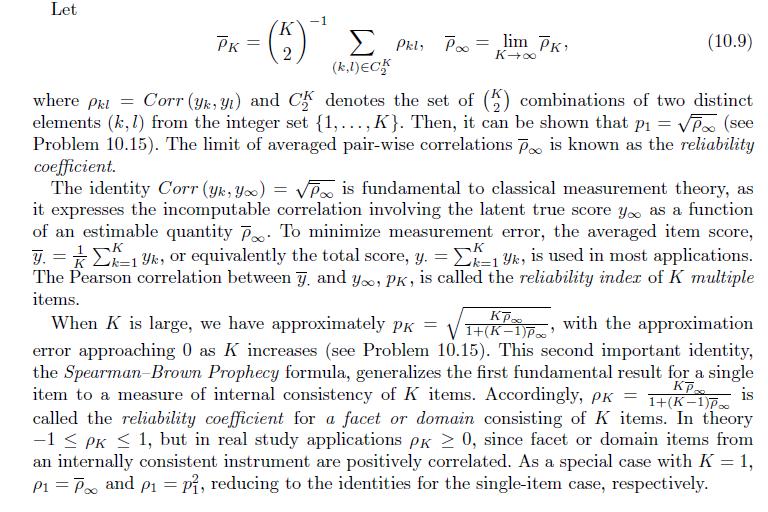
10.4.1 Spearman-Brown Spearman-Brown Rho Internal validity is also known as internal consistency. As noted earlier, it is concerned with the cohesion of a set of items (or questions) when used together to measure a latent construct of interest such as depression, social functioning, or personality. As the total item score is used as a dimensional scale for the latent construct, it is important that such item scores are coherent, or additive. For example, the 36 items of the SF-36 are divided into eight domains: Physical Function (PF), Role-Physical, Bodily Pain, General Health, Vitality, Social Function, Role-Emotional, and Mental-Health. The PF domain has ten items, probing different physical activities such as walking, running, bending, and kneeling (RAND Health). Each item is scored 1, 2, or 3, indicating the respective levels of limitation, "Limited a lot," "Limited a little," and "Not limited at all," when performing each of these activities. For the PF scale to measure the cumulative burden of performing such daily activities, the item scores must be at least positively correlated. One way to ensure such internal consistency is to examine all between-item correlations within the facet or domain. This approach, however, is impractical for facets or domains with a large number of items. In addition, it is difficult to assess the strength of item coherence with a large number of pairwise correlations. A formal framework for assessing internal validity is the domain-sampling model. Under this classic model, a measure of the latent construct of interest is obtained from a random sample of K items from a population of items that this construct underlies. The value of the latent construct is the limit of the averaged item scores y when the number of items approaches infinity, y = lim 19k, akin to the population mean of a random variable. Under the domain-sampling approach, all items share an equal amount of the common core of the construct, i.e., the product-moment (PM) correlation between the kth item and the latent construct yoo, p = Corr (yk, yoo), is a constant independent of any particular item k. This common correlation with yoo is called the reliability index p for a single item.