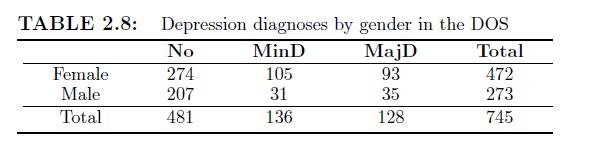
Many measures of association for two-way frequency tables consisting of two ordinal variables are based on the numbers of concordant and discordant pairs. To compute such indices, it is important to count each concordant (discordant) pair exactly once with no misses and repetitions. In Section 2.5.1, we discussed one such counting procedure. Now, let us consider two other alternatives.

(a) For subjects in the cell in the \(i\) th row and \(j\) th column, they will form concordant (discordant) pairs with the subjects in the cells lying to the left of and above (left and below) that cell. The total number of concordant (discordant) pairs is obtained by summing such concordant (discordant) pairs over all the cells in the table. The formula following this approach is given by

\[C=\sum_{i, j} C_{i j}, \quad D=\sum_{i, j} D_{i j}\]

where

\[C_{i j}=2 n_{i j} \sum_{i^{\prime}j^{\prime}} n_{i^{\prime} j^{\prime}}\]

Compute the concordant and discordant pairs for Table 2.8 using this alternative method.

(b) For subjects in the cell in the \(i\) th row and \(j\) th column, they will form concordant (discordant) pairs with the subjects in the cells to the left of and above (left of and below) that cell and to the right of and below (right and above) that cell. The formula following this alternative approach is given by

\[C=\sum_{i, j} C_{i j}, \quad D=\sum_{i, j} D_{i j}\]

where \[\begin{aligned}& C_{i j}=n_{i j}\left(\sum_{i^{\prime}>i, j^{\prime}>j} n_{i^{\prime} j^{\prime}}+\sum_{i^{\prime}), \\& D_{i j}=n_{ij}\left(\sum_{i>i^{\prime}, jj^{\prime}} n_{i^{\prime} j^{\prime}}\right) .\end{aligned}\]
Compute the concordant and discordant pairs for Table 2.8 using this alternative method.

(c) Compare the results.

Transcribed Image Text:

TABLE 2.8: Depression diagnoses by gender in the DOS No MinD Female Male 274 105 MajD 93 Total 472 207 31 35 273 Total 481 136 128 745