A propeller-driven airplane is traveling with a velocity \(V_{1}=200 \mathrm{mph}(294 \mathrm{ft} / \mathrm{s})\). The propeller diameter is \(10 \mathrm{ft}\) and it rotates at \(3000 \mathrm{rpm}\). Figure P12.38 shows a propeller crosssectional profile and the velocity diagrams for a short section of the propeller at a radius of \(3.0 \mathrm{ft}\). The outlet relative velocity \(W_{2}\) is assumed tangent to the propeller at the outlet, so \(\beta_{1}^{\prime}=\beta_{2}=50^{\circ}\). The air density is constant as it flows over the propeller. Assume

Figure P12.38

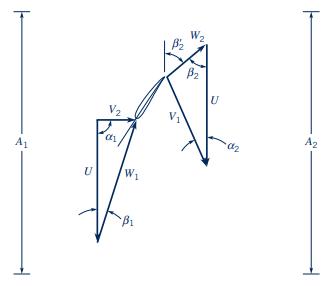
that the flow area for the mass flow rate interacting with this short section of the propeller is the same upstream and downstream (inlet and outlet) from the propeller (i.e., \(A_{1}=A_{2}\) ). Produce the velocity diagram downstream from the propeller by finding \(U, W_{2}, V_{2}\), and \(\alpha_{2}\).

Transcribed Image Text:

A1 U W V W B2 U A2