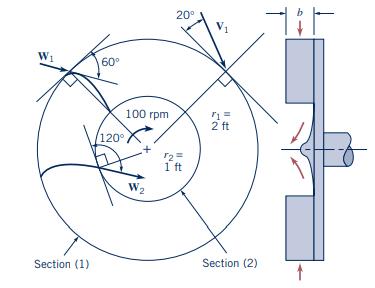
A water turbine wheel rotates at the rate of \(100 \mathrm{rpm}\) in the direction shown in Fig. P12.47. The inner radius, \(r_{2}\), of the blade row is \(1 \mathrm{ft}\), and the outer radius, \(r_{1}\), is \(2 \mathrm{ft}\). The absolute velocity vector at the turbine rotor entrance makes an angle of \(20^{\circ}\) with the tangential direction. The inlet blade angle is \(60^{\circ}\) relative to the tangential direction. The blade outlet angle is \(120^{\circ}\). The flowrate is \(10 \mathrm{ft}^{3} / \mathrm{s}\). For the flow tangent to the rotor blade surface at inlet and outlet, determine an appropriate constant blade height, \(b\), and the corresponding power available at the rotor shaft. Is the shaft power greater or less than the power lost by the fluid? Explain.

Figure P12.47

Transcribed Image Text:

60 120 100 rpm 20 W2 12= 1 ft 2 ft Section (1) Section (2)