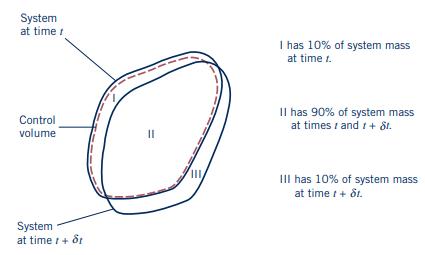
Figure P4.67 illustrates a system and fixed control volume at time \(t\) and the system at a short time \(\delta t\) later. The system temperature is \(T=100^{\circ} \mathrm{F}\) at time \(t\) and \(T=103^{\circ} \mathrm{F}\) at time \(t+\delta t\), where \(\delta t=0.1 \mathrm{~s}\). The system mass, \(m\), is 2.0 slugs, and 10 percent of it moves out of the control volume in \(\delta t=0.1 \mathrm{~s}\). The energy per unit mass \(\tilde{u}\) is \(c_{v} T\), where \(c_{v}=32.0 \mathrm{Btu} / \mathrm{slug} \cdot{ }^{\circ} \mathrm{F}\). The energy \(U\) of the system at any time \(t\) is \(m \tilde{u}\). Use the system or Lagrangian approach to evaluate \(D U / D t\) of the system. Compare this result with the \(D U / D t\) evaluated with that of the material time derivative and flux terms.

Figure P4.67

Transcribed Image Text:

System at time r I has 10% of system mass at timer. Control volume Il has 90% of system mass at times and + 81. System at time / +81 Ill has 10% of system mass at time + St.