Consider the substitutes model in which utility is U(H,z) and the production technology is H=f(x,q). Assume the baseline price of the private input x is p=$10 and that the baseline level of the environmental good is q=2.

(a) Suppose H=x+(1−φ)q, where φ=0.3. Compute the exact willingness to pay for an increase in q from q=2 to q=4.

(b) Suppose H=xφq1−φ, where φ=0.3. Assume we have estimated an ordinary demand for x curve given by x=α−βp+κq, where α=10, β=0.65, and κ=2. What is the baseline level of H? Noting that x is an essential input for the production of H, compute an approximation to the exact willingness to pay for an increase in q from q=2 to q=4.
What is the level of H after the increase in q?

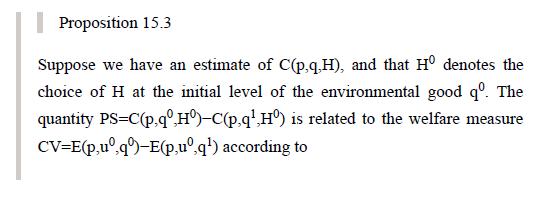
(c) Suppose once again that H=xφq1−φ and x=α−βp+κq, where the parameter values are as given above. Derive the cost function C(p,q,H). Use C(•) to compute a lower bound on the willingness to pay for an increase from q=2 to q=4, using proposition 15.3.

(d) Using the function and parameter values from (c), compute bounds on the willingness to accept for a decrease in q from q=2 to q=1.

Proposition 15.3

Transcribed Image Text:

Proposition 15.3 Suppose we have an estimate of C(p,q,H), and that H denotes the choice of H at the initial level of the environmental good q. The quantity PS=C(p,q,H)-C(p,q,H) is related to the welfare measure CV=E(p,u,q)-E(p,u,q) according to