Suppose we have two polluters that have a hidden characteristic, \(\theta\). The \(\theta\) does not have to be the same for the two firms. Assume that \(\theta\) can take on one of two values: 1 or 2.

These two firms emit pollution, with marginal savings functions equal to

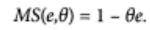
Total savings from polluting for each firm are 

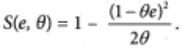
a. Suppose the regulator knew the value of \(\theta\) for each of the firms, \(\theta_{1}\) and \(\theta_{2}\). For all possible combinations of \(\theta_{1}\) and \(\theta_{2}\), what would be the optimal amount of pollution for each firm: \(e_{1}{ }^{*}\left(\theta_{1}, \theta_{2}\right), e_{2}^{*}\left(\theta_{1}{ }^{2}, \theta_{2}\right)\) ?

b. Now assume the regulator does not know \(\theta\) but asks each firm its true \(\theta\). After receiving those reports from each firm, each firm \(i\) will be charged an amount, \(T_{i}\left(e_{i} \theta_{j}\right)\), based on the reported \(\theta_{i}\), the report by the other firm, \(\theta_{ho}\) and actual emissions, \(e_{i}\)

\[T_{i}\left(e_{i}, \theta_{i}\right)=D\left[e_{i}+e_{j}^{*}\left(\theta_{1}, \theta_{2}\right)\right]-S_{j}\left[e_{j}^{*}\left(\theta_{1}, \theta_{2}\right), \theta_{j}\right]\]

where \(i\) and \(j\) are the two firms. The firms know this before they report their values of \(\theta\). Show that it is in the best interest of each firm to tell the truth about \(\theta\) and also to emit the right amount of emissions, \(e^{*}\).

Transcribed Image Text:

MS(e,0)=1-0e.