We consider a non-relativistic particle of mass m in a central potential V(r), where r =√x2 + y2 + z2. We denote the velocity v ≡ ˙r and v2 its square. We study the problem in spherical coordinates (r, θ, φ) defined by

The square of the velocity is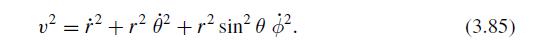

1. Write the Lagrangian of the particle in spherical coordinates.
2. Calculate the conjugate momenta pr , pθ, and pφ.
3. Show that the momentum pφ is equal to the z component of the angular momentum Lz whose expression in Cartesian coordinates is Lz = xpy − ypx.
4. To what invariance law does the conservation of Lz correspond?
5. If the particle is charged and placed in a magnetic field B parallel to the z axis, is the component Lz conserved?

Transcribed Image Text:

x = r sin cos , y = r sin sin o, z = r cos 0. (3.84)