Exercises 8.41 and 8.42 give height and weight data for 18- to 24-year-old U.S. men and women.
Question:
Exercises 8.41 and 8.42 give height and weight data for 18- to 24-year-old U.S. men and women. Use these data to estimate the equation![]()
where Y = weight, X = height, and D = 0 if male, 1 if female.
a. Interpret the coefficient α.
b. Interpret the coefficient β1.
c. Interpret the coefficient β2.
d. Interpret the coefficient β3.
e. Is there a statistically significant difference at the 5 percent level between the effect of height on weight for men and women?
f. Explain why you either agree or disagree with this reasoning: “A person with zero height has zero weight. Therefore, a regression of weight on height must predict zero weight for zero height.”
Exercises 8.41
Table 8.15 shows the average weight (in pounds) for 18- to 24-year-old U.S. men of different heights (measured in inches above 5 feet). Use least squares to estimate the relationship between height (X) and weight (Y).
a. Interpret the estimated coefficient of X.
b. Calculate a 95 percent confidence interval for the coefficient of X.
c. Is the relationship statistically significant at the 5 percent level?
d. The American Heart Association says that the ideal male weight is 110 + 5.0X. How does your fitted line compare to this guideline?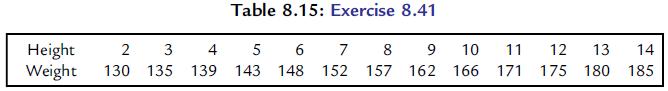
Exercises 8.42
Table 8.16 shows the average weight (in pounds) for 18- to 24-year-old U.S. women of different heights (measured in inches above 5 feet). Use least squares to estimate the relationship between height (X) and weight (Y).
a. Interpret the estimated coefficient of X.
b. Calculate a 95 percent confidence interval for the coefficient of X.
c. Is the relationship statistically significant at the 5 percent level?
d. The American Heart Association says that the ideal female weight is 100 + 5.0X.
How does your fitted line compare to this guideline?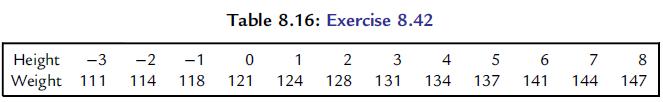
Step by Step Answer:

Essential Statistics Regression And Econometrics
ISBN: 9780123822215
1st Edition
Authors: Gary Smith





