Consider the discrete-time stochastic process where the initial state is often set to , and is an
Question:
Consider the discrete-time stochastic process
![]()
where the initial state is often set to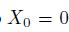 , and
, and 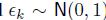 is an element of a sequence of i.i.d. standard normals.
is an element of a sequence of i.i.d. standard normals. 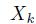 is the state of the system at discrete time
is the state of the system at discrete time 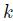 , and it is a continuous random variable, since we add normal variables at each time step. Hence, we have a continuous-state, discrete-time stochastic process. The state is affected by a sequence of shocks
, and it is a continuous random variable, since we add normal variables at each time step. Hence, we have a continuous-state, discrete-time stochastic process. The state is affected by a sequence of shocks 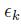 , which are mutually independent, have zero expected value, and are also independent on the current state. Hence, the shocks are unpredictable. Note that we insist on adding a shock indexed by
, which are mutually independent, have zero expected value, and are also independent on the current state. Hence, the shocks are unpredictable. Note that we insist on adding a shock indexed by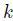 to a state variable indexed by
to a state variable indexed by 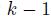 , to emphasize the nature of the shocks, which are often referred to as innovations in econometric parlance. Their independence on the past is related to the efficient market hypothesis. This kind of process is called a random walk. As we shall see, there is a corresponding process in continuous time, called standard Wiener process, which plays a key role in financial modeling.By unfolding Eq. (11.1) recursively, we find
, to emphasize the nature of the shocks, which are often referred to as innovations in econometric parlance. Their independence on the past is related to the efficient market hypothesis. This kind of process is called a random walk. As we shall see, there is a corresponding process in continuous time, called standard Wiener process, which plays a key role in financial modeling.By unfolding Eq. (11.1) recursively, we find
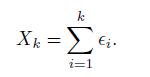
Given the mutual independence and the normality of the driving shocks, we easily find the unconditional distribution of the state,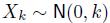 . Please note that the variance is
. Please note that the variance is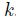 . Hence, the standard deviation is
. Hence, the standard deviation is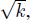 i.e., it scales with the square root of (discrete) time. It is also easy to find conditional distributions. Conditional on the value
i.e., it scales with the square root of (discrete) time. It is also easy to find conditional distributions. Conditional on the value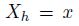 at time
at time 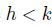 we have
we have
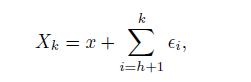
and
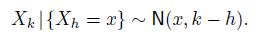
This is an example of a Gaussian process, since the joint distribution of the random variables 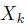 is normal.
is normal.
Data FromEq. (11.1)
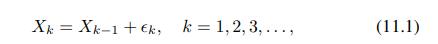
Step by Step Answer:

An Introduction To Financial Markets A Quantitative Approach
ISBN: 9781118014776
1st Edition
Authors: Paolo Brandimarte





