In the setting of Proposition 4.27, show that, if, for any \(i=1, \ldots, I\), the utility function \(u^{i}: \mathbb{R}^{2} \rightarrow \mathbb{R}\) is strictly increasing in the first argument and non-decreasing in the second argument, then the existence of an optimal portfolio excludes the existence of arbitrage opportunities of the second kind.

Data From Proposition 4.27

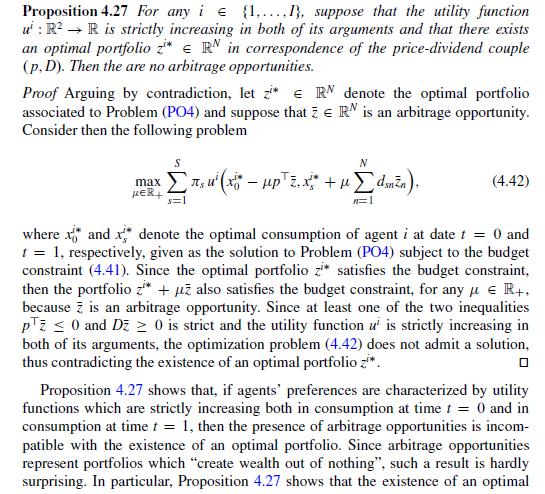
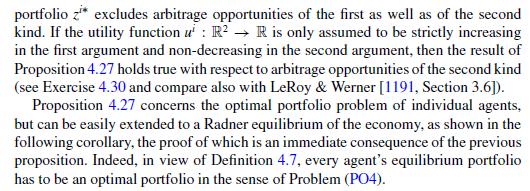
Transcribed Image Text:

Proposition 4.27 For any i = {1,..., suppose that the utility function u: R R is strictly increasing in both of its arguments and that there exists an optimal portfolio zi e RN in correspondence of the price-dividend couple (p,D). Then the are no arbitrage opportunities. Proof Arguing by contradiction, let zi associated to Problem (PO4) and suppose that Consider then the following problem max R+ RN denote the optimal portfolio RN is an arbitrage opportunity. N (x - up zx * + dmin), == (4.42) where and denote the optimal consumption of agent i at date t = 0 and t = 1, respectively, given as the solution to Problem (PO4) subject to the budget constraint (4.41). Since the optimal portfolio z satisfies the budget constraint, then the portfolio z* + u also satisfies the budget constraint, for any R+, because is an arbitrage opportunity. Since at least one of the two inequalities pz0 and Dz 0 is strict and the utility function u is strictly increasing in both of its arguments, the optimization problem (4.42) does not admit a solution, thus contradicting the existence of an optimal portfolio zi*. Proposition 4.27 shows that, if agents' preferences are characterized by utility functions which are strictly increasing both in consumption at time t = 0 and in consumption at time t = 1, then the presence of arbitrage opportunities is incom- patible with the existence of an optimal portfolio. Since arbitrage opportunities represent portfolios which "create wealth out of nothing", such a result is hardly surprising. In particular, Proposition 4.27 shows that the existence of an optimal