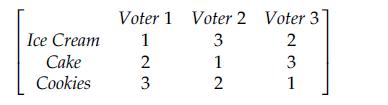
Three traders are at lunch and must decide on a dessert that they will share. Consider the following table, which compares trader preferences for three types of desserts, all ranked according to preferences one through three for each trader. Individual trader preferences are rational. There are three possible desserts, and thus three possible preference rankings. The traders have determined that if they vote on which dessert they will order, each of the three desserts will receive one vote, resulting in a tie. Thus, the traders decided to hold pairwise votes on combinations of two desserts. That is, they will eliminate desserts one at a time in three votes, with the winning dessert being matched against the next dessert. Thus, ice cream will be matched against cake, and then against cookies and then cookies against cake. Demonstrate that a pairwise vote (one dessert option against another) on any combination of two desserts would ultimately result in a “social” selection that will violate the principle of transitivity.

Transcribed Image Text:

Ice Cream Cake Cookies Voter 1 Voter 2 Voter 37 1 3 2 3 1 23 12