Consider the Clairaut equation for which g(y') = -1/4 (y') 2 in Eq. (37). Show that the
Question:
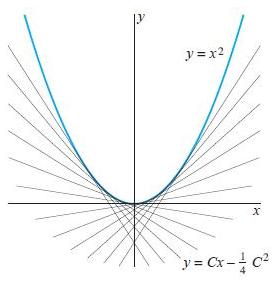
Consider the Clairaut equation
![]()
for which g(y') = -1/4 (y')2 in Eq. (37). Show that the line
![]()
is tangent to the parabola
![]()
Explain why this implies that y = x2 is a singular solution of the given Clairaut equation. This singular solution and the one-parameter family of straight line solutions are illustrated in Fig. 1.6.10.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Differential Equations And Linear Algebra
ISBN: 9780134497181
4th Edition
Authors: C. Edwards, David Penney, David Calvis
Question Posted:





