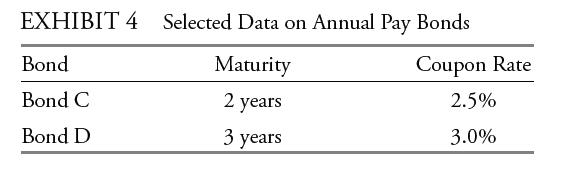
Based on Exhibits 4 and 7, the present value of Bond D’s cash flows following Path 2 i  closest to:

A. 97.0322.

B. 102.8607.

C. 105.8607.

Meredith Alvarez is a junior fixed-income analyst with Canzim Asset Management. Her supervisor, Stephanie Hartson, asks AlvarezA to review the asset price and payoff data shown in Exhibit 1 to determine whether an arbitrage opportunity exists.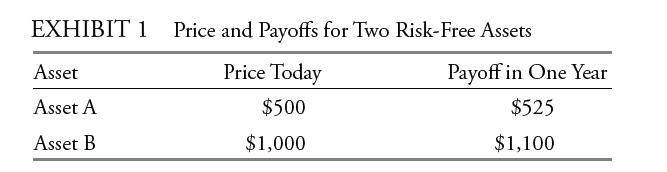

Hartson also shows Alvarez data for a bond that trades in three different markets in the same currency. These data appear in Exhibit 2.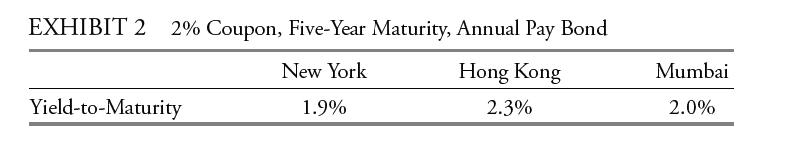

Hartson asks Alvarez to value two bonds (Bond C and Bond D) using the binomial tree in Exhibit 3. Exhibit 4 presents selected data for both bonds.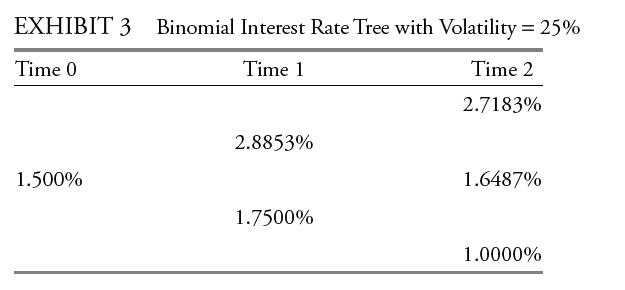

Hartson tells Alvarez that she and her peers have been debating various viewpoints regarding the conditions underlying binomial interest rate trees. The following statements were made in the course of the debate.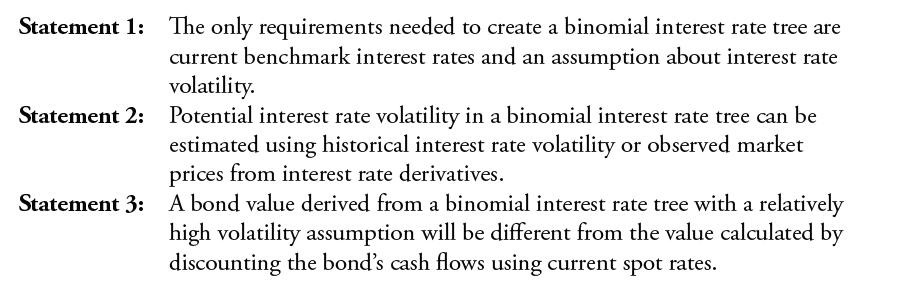

Based on data in Exhibit 5, Hartson asks Alvarez to calibrate a binomial interest rate tree starting with the calculation of implied forward rates shown in Exhibit 6.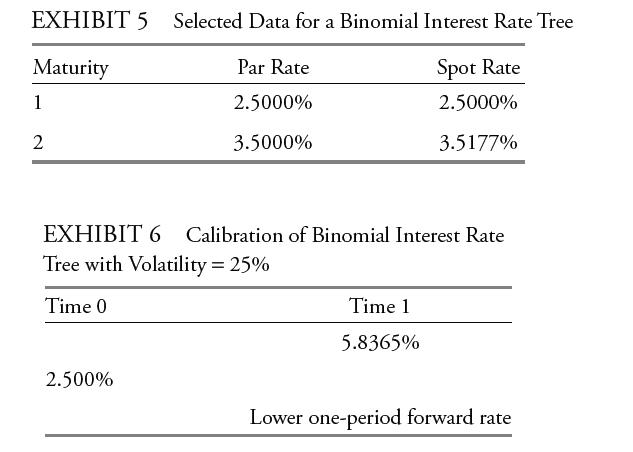

Hartson mentions pathwise valuations as another method to value bonds using a binomial interest rate tree. Using the binomial interest rate tree in Exhibit 3, Alvarez calculates the possible interest rate paths for Bond D shown in Exhibit 7.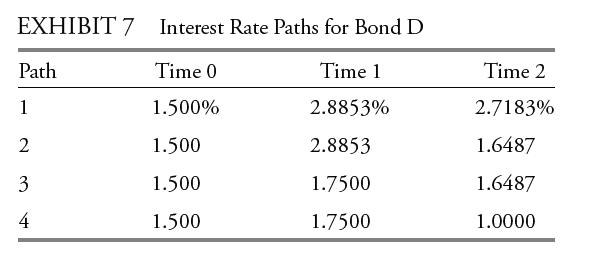

Before leaving for the day, Hartson asks Alvarez about the value of using the Monte Carlo method to simulate a large number of potential interest rate paths to value a bond. Alvarez makes the following statements.

Transcribed Image Text:

EXHIBIT 1 Price and Payoffs for Two Risk-Free Assets Price Today $500 $1,000 Asset Asset A Asset B Payoff in One Year $525 $1,100