In an irrotational region of flow, we write the velocity vector as the gradient of the scalar
Question:
In an irrotational region of flow, we write the velocity vector as the gradient of the scalar velocity potential function,
V(vector) in cylindrical coordinates, (r, u, z) and (ur, uθ, uz), are
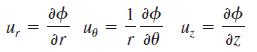
From Chap. 9, we also write the components of the vorticity vector in cylindrical coordinates as
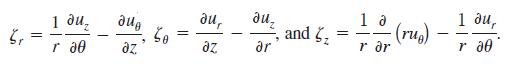
Substitute the velocity components into the vorticity components to show that all three components of the vorticity vector are indeed zero in an irrotational region of flow.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
In an irrotational region of flow the velocity vector is Now the components of in cylindric...View the full answer
Answered By
Gabriela Rosalía Castro
I have worked with very different types of students, from little kids to bussines men and women. I have thaught at universities, schools, but mostly in private sessions for specialized purpuses. Sometimes I tutored kids that needed help with their classes at school, some others were high school or college students that needed to prepare for an exam to study abroud. Currently I'm teaching bussiness English for people in bussiness positions that want to improve their skills, and preparing and ex-student to pass a standarized test to study in the UK.
1+ Reviews
10+ Question Solved
Related Book For 
Fluid Mechanics Fundamentals And Applications
ISBN: 9780073380322
3rd Edition
Authors: Yunus Cengel, John Cimbala
Question Posted:
