In the derivation of the Bernoulli equation for regions of inviscid flow, we rewrite the steady, incompressible Euler equation into a form showing that the gradient of three scalar terms is equal to the velocity vector crossed with the vorticity vector, noting that z is vertically upward,

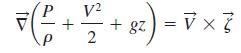
We then employ some arguments about the direction of the gradient vector and the direction of the cross product of two vectors to show that the sum of the three scalar terms must be constant along a streamline. In this problem you will use a different approach to achieve the same result. Namely, take the dot product of both sides of the Euler equation with velocity vector V(vector) and apply some fundamental rules about the dot product of two vectors. Sketches may be helpful.

Transcribed Image Text:

TD V² 2 2xA= (28+