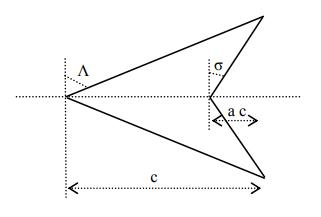
The delta wing given in Fig. 8.59 has the supersonic lift line slope, according to Puckett and Stewart, as follows

\[
\begin{aligned}
& \frac{d C_{l}}{d \alpha}=\left(2 \pi \cot \Lambda / E\left(m^{\prime}\right)\right) H(a) \\
& \quad H(a)=\frac{2}{\pi}\left[\frac{a}{1+a}+\frac{1-a}{\left(1-a^{2}\right)^{3 / 2}} \cos ^{-}(-a)\right]
\end{aligned}
\]

What is the lift coefficient of the wing given in Example 8.1 having a leading edge with \(35^{\circ}\), and a trailing edge with \(75^{\circ}\) sweep?

Fig. 8.59

Example 8.1

Euler Equation numerical solver for a delta wing with \(75^{\circ}\) sweep at \(M=1.95\) and \(10^{\circ}\) angle of attack gives the normal force coefficient as \(C_{N}=0.295\) (Murman and Rizzi 1986). Find the normal force coefficient with: 

(i) incompressible potential flow 

(ii) supersonic potential flow, 

(iii) Polhamus theory.

Transcribed Image Text:

C a c.