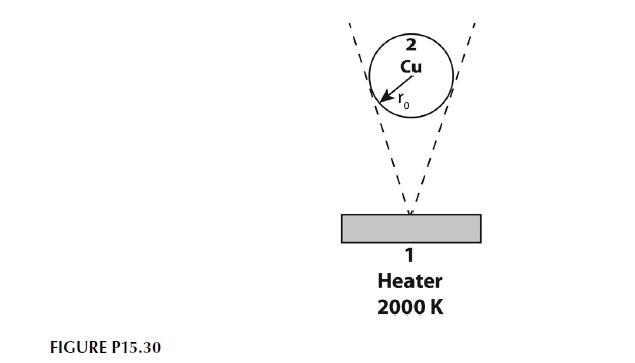
A copper ball bearing of radius \(r_{0}=0.01 \mathrm{~m}\) is exposed to a small heat source that operates at a temperature of \(2000 \mathrm{~K}\) and has an emissivity of 0.75 . The situation is shown in Figure P15.30 with the copper sphere being \(0.05 \mathrm{~m}\) away from the surface. The copper needs to be heated for tempering and must reach a temperature of \(500 \mathrm{~K}\) from \(98 \mathrm{~K}\).

a. Derive the differential equation governing the temperature of the sphere. You may assume the emissivity of the copper does not depend on temperature.

b. How long does it take for the copper to reach its tempering temperature? You may want to solve the problem using what is called a radiation heat-transfer coefficient, \(h_{\mathrm{rad}}\) :

\[q_{\text {net }}=\frac{\sigma^{r}\left(T_{1}^{4}-T_{2}^{4}\right)}{\sum R}=\underbrace{\frac{\sigma^{r}\left(T_{1}^{2}+T_{2}^{2}\right)\left(T_{1}+T_{2}\right)}{\sum_{R} R}}_{\boldsymbol{h}_{\text {rad }} \boldsymbol{A}}\left(T_{1}-T_{2}\right)\]

Transcribed Image Text:

FIGURE P15.30 2 Cu 0 1 Heater 2000 K