In Section 10.2.2 we calculated the velocity field for flow past a stationary sphere. Such a sphere
Question:
In Section 10.2.2 we calculated the velocity field for flow past a stationary sphere. Such a sphere experienced a buoyant force but no lift. To generate lift, we need to rotate the sphere. We can calculate the velocity profile for the rotating sphere by changing the boundary condition on the velocity at the surface of the sphere. For a sphere rotating about the \(\theta\)-direction at a rate of \(B\) revolutions per second (Hint: \(v_{\theta}=2 \pi r_{o} B \sin \theta\) ):
a. What are the boundary conditions on the sphere surface?
b. Solve for the stream function.
c. What are the \(r\) and \(\theta\) velocity components?
d. Substitute the velocity into the momentum equations, determine an expression for the pressure (many pages of algebra).
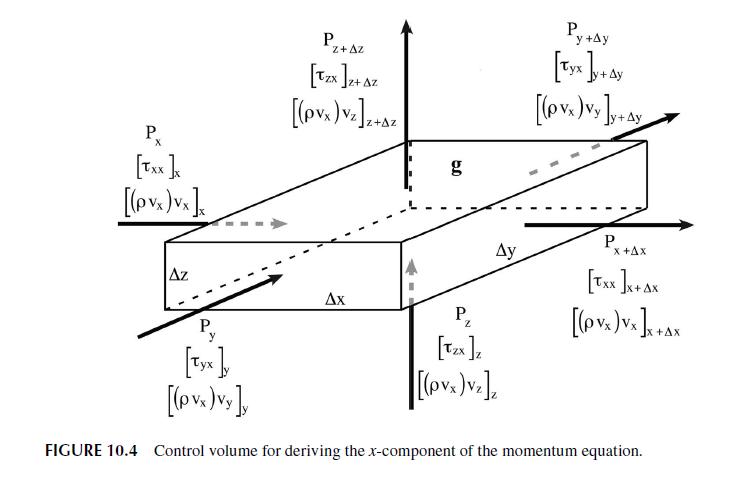
e. Using the results of part (d) to integrate the normal component of the pressure, and by comparing with the stationary solution, isolate the lift component of the normal force (many more pages of algebra).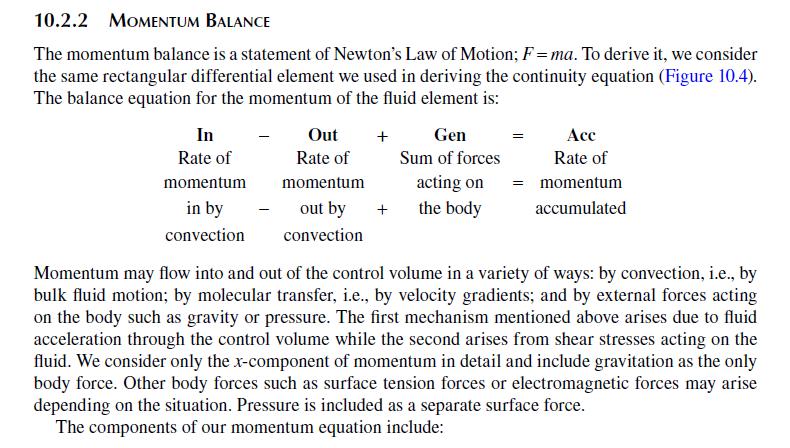
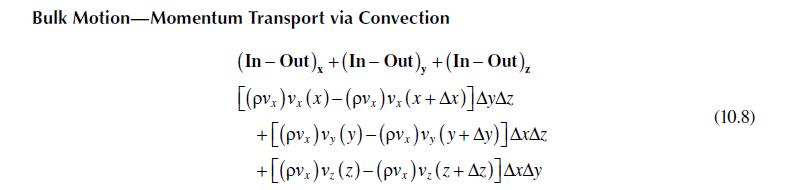
Step by Step Answer:






