We are often interested in modeling the growth and spread of a population of bacteria. While we
Question:
We are often interested in modeling the growth and spread of a population of bacteria. While we often have a good idea of bacterial reproduction rates, getting a handle on how fast they spread is equally important. Segel et al. developed a simple way to measure the motility/diffusion coefficient for a bacterial population using a capillary tube experiment [14]. Imagine a capillary tube of area, A, with one end sealed, initially filled with pure growth media. At time \(t=0\), the open end of the tube is placed in a bacterial suspension containing \(N_{0}\) cells \(/ \mathrm{m}^{3}\). The tube is removed from the suspension at a time \(t=T\), and the number of bacteria in the tube is counted and determined to be \(N\) cells. The mobility can be calculated from:
\[\mu_{\text {cells }}=\frac{\pi N_{\text {tot }}^{2}}{4 N_{0}^{2} A^{2} T}\]
based on the governing differential equation that assumed only diffusion occurring in the capillary and that the cells climb only a short distance up the capillary tube in the time, \(T\).
\[\frac{\partial N}{\partial t}=\mu_{\text {cell }} \frac{\partial^{2} N}{\partial x^{2}}\]
a. Given that the mobility has units of a diffusivity, is the equation dimensionally correct?
b. At the open end of the tube, what is the flux of bacteria?
c. Based on your answer to part (b), derive Segel et al.'s result for the mobility.
d. Suppose the radius of the capillary tube is \(100 \mu \mathrm{m}\), the bacterial suspension at the tube opening is \(7 \times 10^{13}\) cells \(/ \mathrm{m}^{3}\) and the time history of the cell population in the tube is:
what is the value of \(\mu_{\text {cell }}\) for each time?
e. Assuming we only measure cell numbers at \(T=600 \mathrm{~s}\), what is the value for \(\mu_{\text {cell }}\) given the data below?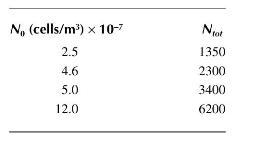
Step by Step Answer:






