A sixth-grade teacher uses a new method of teaching mathematics to her students, one she believes will increase their level of mathematical ability. To assess their mathematical ability, she administers a standardized test (the Test of Computational Knowledge [TOCK]). Scores on this test can range from a minimum of 20 to a possible maximum of 80. The TOCK scores for the 20 students are listed below: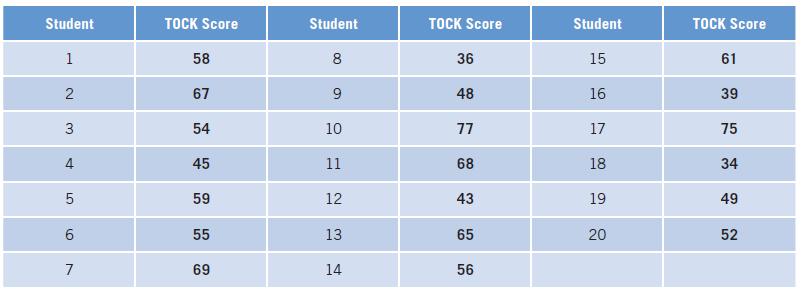

To interpret their level of performance on the test, she wishes to compare their mean with the hypothesized population mean (μ) of 50 for sixth graders in her state.

a. Calculate the mean (X ) and standard deviation (s) of the TOCK scores.

b. State the null and alternative hypotheses (H₀ and H₁) (allow for the possibility that the new teaching method may, for some reason, lower students’ mathematical ability).

c. Make a decision about the null hypothesis.

(1) Calculate the degrees of freedom (df).
(2) Set alpha (α), identify the critical values (draw the distribution), and state a decision rule.
(3) Calculate a statistic: t-test for one mean.
(4) Make a decision whether to reject the null hypothesis.
(5) Determine the level of significance.

d. Draw a conclusion from the analysis.

e. Relate the result of the analysis to the research hypothesis.

Transcribed Image Text:

Student TOCK Score Student TOCK Score Student TOCK Score 1 58 8 36 15 61 2 67 9 48 16 39 3 54 10 77 17 75 4 45 11 68 18 34 5 59 12 43 19 49 6 55 13 65 20 52 52 7 69 14 56