Consider a container exposed to a saturated vapor, (T_{text {sat }}), having a cold bottom surface, (T_{s}
Question:
Consider a container exposed to a saturated vapor, \(T_{\text {sat }}\), having a cold bottom surface, \(T_{s} Assuming a linear temperature distribution for the liquid, perform a surface energy balance on the liquid-vapor interface to obtain the following expression for the growth rate of the liquid layer: \[\delta(t)=\left[\frac{2 k_{l}\left(T_{\text {sat }}-T_{s}\right)}{ho_{l} h_{f g}} t\right]^{1 / 2}\] Calculate the thickness of the liquid layer formed in \(1 \mathrm{~h}\) for a \(200-\mathrm{mm}^{2}\) bottom surface maintained at \(80^{\circ} \mathrm{C}\) and exposed to saturated steam at \(1 \mathrm{~atm}\). Compare this result with the condensate formed by a vertical plate of the same dimensions for the same period of time.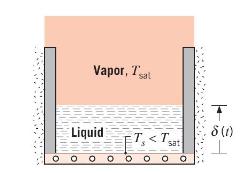
Step by Step Answer:

Fundamentals Of Heat And Mass Transfer
ISBN: 9781119220442
8th Edition
Authors: Theodore L. Bergman, Adrienne S. Lavine





