During the experiment described in Problem 2.2, the air velocity was measured at (10 mathrm{~m} / mathrm{s}).
Question:
During the experiment described in Problem 2.2, the air velocity was measured at \(10 \mathrm{~m} / \mathrm{s}\). Estimate the mass-transfer coefficient predicted by equation (2-64) and compare it to the value measured experimentally. The following data for naphthalene might be needed: \(T_{b}=491.1 \mathrm{~K}, V_{c}=413 \mathrm{~cm}^{3} / \mathrm{mol}\).
Data From Problem 2.2:-
In a laboratory experiment, air at \(347 \mathrm{~K}\) and \(1 \mathrm{~atm}\) is blown at high speed around a single naphthalene \(\left(\mathrm{C}_{10} \mathrm{H}_{8}\right)\) sphere, which sublimates partially. When the experiment begins, the diameter of the sphere is \(2.0 \mathrm{~cm}\). At the end of the experiment, 14.32 min later, the diameter of the sphere is \(1.85 \mathrm{~cm}\).
(a) Estimate the mass-transfer coefficient, based on the average surface area of the particle, expressing the driving force in terms of partial pressures. The density of solid naphthalene is \(1.145 \mathrm{~g} / \mathrm{cm}^{3}\) and its vapor pressure at \(347 \mathrm{~K}\) is \(670 \mathrm{~Pa}\) (Perry and Chilton, 1973).
(b) Calculate the mass-transfer coefficient, for the driving force in terms of molar concentration.
Data From Equation 2-64:-
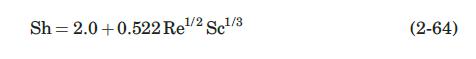
Step by Step Answer:






