Consider a risky asset valued \(S_{0}=\$ 4\) at time \(t=0\), and taking only two possible values \(S_{1} \in\{\$ 5, \$ 2\}\) at time \(t=1\), and the claim payoff 

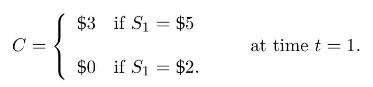
We assume that the issuer charges \(\$ 1\) for the option contract at time \(t=0\).

a) Compute the portfolio allocation \((\xi, \eta)\) made of \(\xi\) stocks and \(\$ \eta\) in cash, so that:
i) the full \(\$ 1\) option price is invested into the portfolio at time \(t=0\), and ii) the portfolio reaches the \(C=\$ 3\) target if \(S_{1}=\$ 5\) at time \(t=1\).

b) Compute the loss incurred by the option issuer if \(S_{1}=\$ 2\) at time \(t=1\).

Transcribed Image Text:

C $3 if S = $5 $0 if S = $2. at time t = 1.