Consider an underlying asset price process (left(S_{t} ight)_{t in mathbb{R}_{+}})modeled as (d S_{t}=(mu-delta) S_{t} d t+sigma S_{t}

Consider an underlying asset price process \(\left(S_{t}\right)_{t \in \mathbb{R}_{+}}\)modeled as \(d S_{t}=(\mu-\delta) S_{t} d t+\sigma S_{t} d B_{t}\), where \(\left(B_{t}\right)_{t \in \mathbb{R}_{+}}\)is a standard Brownian motion and \(\delta>0\) is a continuous-time dividend rate.

a) Write down the self-financing condition for the portfolio value \(V_{t}=\xi_{t} S_{t}+\eta_{t} A_{t}\) with \(A_{t}=A_{0} \mathrm{e}^{r t}\), assuming that all dividends are reinvested.

b) Derive the Black-Scholes PDE for the function \(g_{\delta}(t, x, y)\) such that \(V_{t}=g_{\delta}\left(t, S_{t}, \Lambda_{t}\right)\) at time \(t \in[0, T]\).

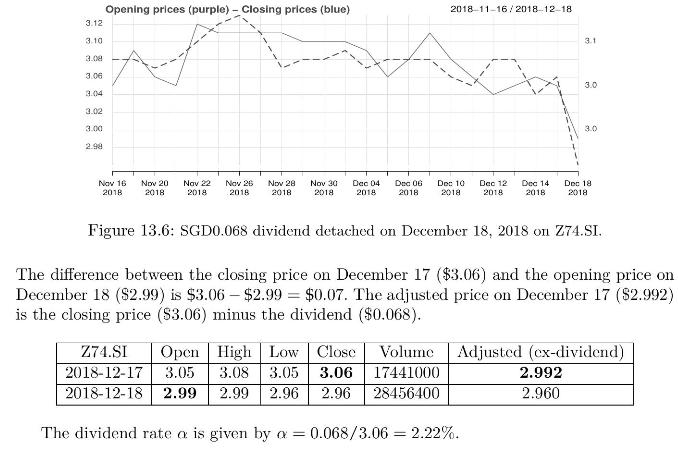
Transcribed Image Text:

1 3 getDividends('Z74.SI" from "2018-01-01", to="2018-12-31",src="yahoo") getSymbols("Z74.SI", from="2018-11-16', to='2018-12-19",arc="yahoo") Tchart theme(); TScol$line.col