Recall that the Fourier series representation of a periodic function is

\(F=\frac{a_{0}}{2}+\sum_{i=1}^{\infty}\left(a_{i} \cos \omega_{i} t+b_{i} \sin \omega_{i} t\right)\)

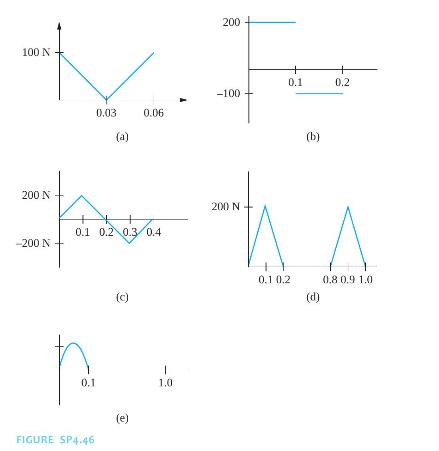
Describe which of the Fourier coefficients \(\left(a_{0}, a_{i}, b_{i}\right.\), or none) are zero for each of the functions (illustrated over one period) shown in Figure SP4.46.

Transcribed Image Text:

100 N 200 0.1 0.2 -100 0.03 0.06 (a) 200 N + 0.1 0.2 0.3 0.4 -200 N 0.1 (c) FIGURE SP4.46 (e) 1 1.0 200 N+ (b) 0.1 0.2 (d) 0.8 0.9 1.01