Use a three-element, finite-element model to approximate the lowest natural frequency and its corresponding mode shape for the system of Figure P11.15.

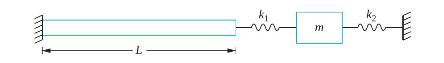
\[ \begin{array}{ll} E=200 \times 10^{9} \mathrm{~N} / \mathrm{m}^{2} & m=1.2 \mathrm{~kg} \\ A=3.5 \times 10^{-5} \mathrm{~m}^{2} & k_{1}=2 \times 10^{6} \mathrm{~N} / \mathrm{m} \\ L=2.5 \mathrm{~m} & k_{2}=1.4 \times 10^{6} \mathrm{~N} / \mathrm{m} \\ ho=7000 \mathrm{~kg} / \mathrm{m}^{3} & \end{array} \]

FIGURE P11.15

L k m