Circular jets of liquid are notoriously unstable. The breakup of one into drops may be observed by placing a finger under a thin stream of water from a faucet and noticing the rapid series of impacts at a certain distance from the nozzle. The objective is to explore why liquid cylinders break up and to estimate how fast that occurs in a water jet. The jet radius is R and its velocity in a lab reference frame is U, as shown in Fig. P1.7(a). The enlargement in Fig. P1.7(b) shows the breakup process in a reference frame moving with the jet. Transforming a segment of length L into a sphere of radius a involves relative velocities on the order of v, which is an unknown. To form the drop, a typical element of liquid must travel a distance in the jet reference frame that is a few times R.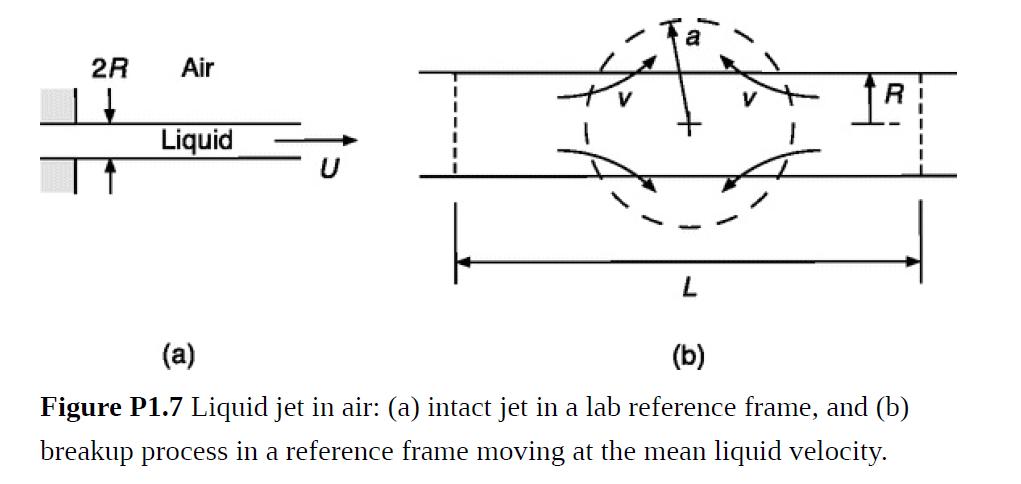

(a) In that γ is the energy per unit area of interface and systems seek lower energy states, a liquid cylinder will be unstable if it can reduce its surface area. Show that the cylinder–sphere transformation in Fig. P1.7(b) will reduce the area if L⁄R > 9⁄2. This differs somewhat from the actual criterion for instability, because small deformations must reduce the area immediately if the shape change is to continue; the cylinder does not “know” at the outset that it can become a sphere.

(b) For low-viscosity liquids such as water, L⁄R = 9.0 results in the fastest breakup (Eggers and Villermaux, 2008) and is what is usually seen. Accordingly, what drop size would you expect from the breakup of a water jet with R = 1 mm?

(c) Jet breakup due to surface tension is resisted by inertia and viscosity. If solely inertia were involved, the time for breakup would be t_is, the inertial– surface-tension time scale. The corresponding velocity scale is v = R/t_is. By equating the inertial force scale to that for surface tension, derive an expression for t_is. Evaluate t_is for a water jet with R = 1 mm.

(d) The viscous–surface-tension time scale (t_vs) is calculated similarly. Show that the ratio of time scales is

and that Oh, the Ohnesorge number, is small for the water jet of part (c). This implies that inertia slows the breakup much more than does viscosity. Accordingly, the actual time for the water jet to break into drops is roughly t_is, and the jet will travel a distance of approximately Ut_is before drops form.

Transcribed Image Text:

2R ↓ Air Liquid (a) (b) Figure P1.7 Liquid jet in air: (a) intact jet in a lab reference frame, and (b) breakup process in a reference frame moving at the mean liquid velocity.