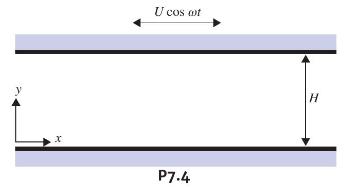
Fluid fills the space between two parallel plates. The differential equation that describes the instantaneous fluid velocity for unsteady flow with the fluid moving parallel to the walls is

\[ho \frac{\partial u}{\partial t}=\mu \frac{\partial^{2} u}{\partial y^{2}}\]

The lower plate is stationary and the upper plate oscillates in the \(x\)-direction with a frequency \(\omega\) and an amplitude in the plate velocity of \(U\). Use the characteristic dimensions to normalize the differential equation and obtain the dimensionless groups that characterize the flow.

Transcribed Image Text:

x U cos mot P7.4 H