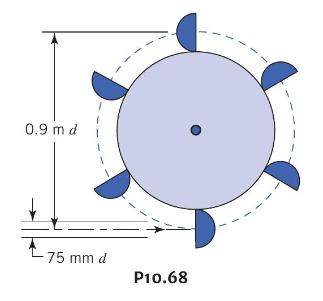
The velocity of the water jet driving this impulse turbine is \(45 \mathrm{~m} / \mathrm{s}\). The jet has a \(75-\mathrm{mm}\) diameter. After leaving the buckets the absolute velocity of the water is observed to be \(15 \mathrm{~m} / \mathrm{s}\) in a direction \(60^{\circ}\) to that of the original jet. Calculate the mean tangential force exerted by the jet on the turbine wheel and the speed (rpm) of the wheel.

Transcribed Image Text:

0.9 m d - 75 mm d P10.68