In life testing, we are sometimes interested in establishing tolerance limits for the life of a component in particular, we may be interested in a one-sided tolerance limit \(t^{*}\), for which we can assert with a \((1-\alpha) 100 \%\) confidence that at least \(100 \cdot P\) percent of the components have a life exceeding \(t^{*}\). Using the exponential model, it can be shown that

\[t^{*}=\frac{-2 T_{r}(\ln P)}{\chi_{\alpha}^{2}}\]

where \(T_{r}\) is as defined on page 511, and the value of \(\chi_{a}^{2}\) is to be obtained from Table \(5 \mathrm{~W}\) with \(2 r\) degrees of freedom.

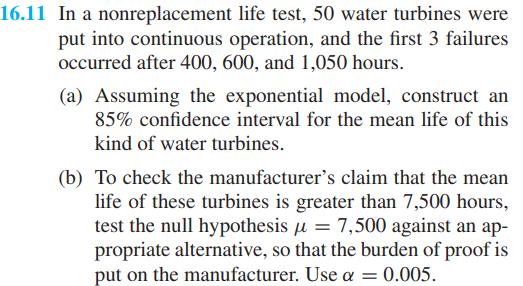
(a) Using the data of Exercise 16.11, establish a lower tolerance limit for which one can assert with \(99 \%\) confidence that it is exceeded by at least \(75 \%\) of the lifetimes of water turbines.

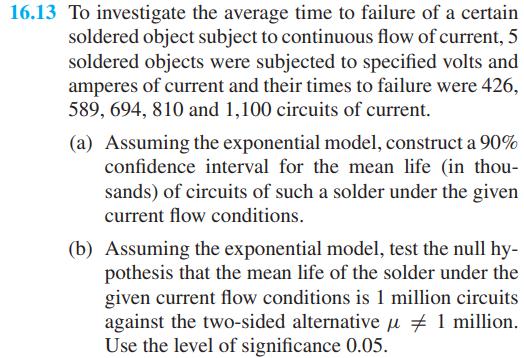
(b) Using the data of Exercise 16.13, establish a lower tolerance limit for which one can assert with \(95 \%\) confidence that it is exceeded by at least \(80 \%\) of the lifetimes of a given solder.

Data From Page 511

Data From Exercise 16.11

Data From Exercise 16.13

Data from Table 5W

Transcribed Image Text:

EXAMPLE 3 Obtaining a confidence interval for mean life Suppose that 50 units are placed on life test (without replacement) and the test is to be truncated after r = 10 of them have failed. We shall suppose, furthermore, that the first 10 failure times are 65, 110, 380, 420, 505, 580, 650, 840, 910, and 950 hours. Estimate the mean life of the component, and its failure rate, and calculate a 90% confidence interval for u.