Let A, B, and C be independent normal N(1, 1) random variables Let X(t), t 0,) be defined as Also, let Y (t), t 0,) be defined as Find R XY (t 1 , t 2 ) and C XY (t 1 , t 2 ), for t 1 , t 2 0,) X(t) A Bt, for all t 0, ) ...

First note that Similarly xt EXt EAEBt 1t for all t 0 uYtEYt EA ECt 1t ...

Let A, B, and C be independent normal N(1, 1) random variables. Let {X(t), t [0,)}

Question:

Let A, B, and C be independent normal N(1, 1) random variables. Let {X(t), t ∈ [0,∞)} be defined as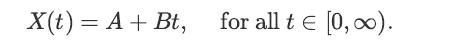 X(t) = A + Bt, for all t = [0, ).

Also, let {Y (t), t ∈ [0,∞)} be defined as

Find R_XY (t₁, t₂) and C_XY (t₁, t₂), for t₁, t₂ ∈ [0,∞).

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Introduction To Probability Statistics And Random Processes

ISBN: 9780990637202

1st Edition

Authors: Hossein Pishro-Nik

See More Books

Question Posted: Nov 01, 2023 01:42 PM

See More Questions

Let A, B, and C be independent random variables, uniformly distributed on (0, 1). What is the probability that Ax2 + Bx + C has real roots? (If X ~ uniform(0,1), then - log X ~ exponential. The sum...
Let B and C be n n matrices with the property Bx = Cx for all x Rn. Show that B = C.
Let B and C be bases for P2. If B = {x, 1 + x, 1 -x + x2} and the change-of-basis matrix from B to C is Find C. 011 101
Consider the following propositions: p: 2 is the smallest prime q: 6 is a perfect square Represent the proposition "2 is the smallest prime but 6 is not a perfect square" using logical connectives....
How can information produced by a job order costing system assist managers in operating their firms more efficiently?
Keeper Corporations income statement for the year ended June 30, 2014, and its comparative balance sheets for June 30, 2014 and 2013 follow. Keeper Corporation Income Statement For the Year Ended...
How has it been shown that suggestion can infl uence peoples memories for early events in their lives? lo1
Five years ago, to respond to cost-cutting pressure during a weak economy, your company decided to close five sales offices employing five people each. Currently your company employs independent...
Sonia and Ted are married, have three young children, and file a joint return in 2021. If they have adjusted gross income (AGI) of $100,000 and itemized deductions of $12,000, what is their taxable...
Let X(t) be a Gaussian process such that for all t > s 0 we have X(t) X(s) N (0, t s). Show that X(t) is mean-square continuous at any time t 0.
Find the correlation functions and covariance functions for the random processes given in Examples 10.1 and 10.2. Examples 10.1 You have 1000 dollars to put in an account with interest rate R,...
a. The convention committee is considering Portland, Oregon; New Orleans, Louisiana; and Phoenix, Arizona. b. The convention committee is considering Portland, Oregon, New Orleans, Louisiana, and...
In your reflection journal please list your order - 'most efficient' mediums at the top, 'least efficient' at the bottom. (for eg. social media, display ads, etc)Then, in five hundred words or more,...
Energy prices and global warming are discussed daily in the news as environmental impact of e-waste is just beginning to be recognized. Sustainability and corporate social responsibility need to be...
3. A Channel section is connected to a 10mm gusset plate with 20mm- diameter bolts as shown in the figure. The connecting member is subjected to dead load and live load only. The pitch distance,...
Create your own privacy philosophy. This should cover the policies you will use for email, texting, social media, and internet usage. Consider what information is being collected anout you in each...
1. What future markets might be attractive to Carrefour and which mode of operation would be preferable? How important is the theoretical concept of psychological distance? 2. Corporate...
Joy's Frozen Yogurt shops have enjoyed rapid growth in northeastern states in recent years. From the analysis of joy's various outlets, it was found that die demand curve follows this pattern: Q =...
Consider the circuit of Fig. 7.97. Find v0 (t) if i(0) = 2 A and v(t) = 0. 1 3 ett)
Which tax form is used by the following individuals? a. Anita is single, age 68, and has a salary of $22,000 and interest of $300. b. Betty owns an apartment complex that produced rental income of...
a. If a taxpayer must travel away from his or her home in order to obtain medical care, which en route costs, if any, are deductible as medical expenses? b. Are there any limits imposed on the...
What are the rules dealing with the deductibility of the cost of meals and lodging incurred while away from home in order to receive medical treatment as an outpatient?
What would be some pros and cons of using acutal versus capacity cost driver rates and vice versus. this is the question i am ultimately answering, "Which cost driver rates should be used: actual or...
The Auditors Responsibility with Going Concerns Read The auditors consideration of an entitys ability to continue as a going concern. Based on the reading, analyze the auditors responsibility to...
On September 1, 20X1, the account balances of Rand Equipment Repair, Inc. were as follows. Sept. 8 Paid 1,300 for salaries due employees, of which 500 is for August. 9 Shareholders invested 13,000...

Let A, B, and C be independent normal N(1, 1) random variables. Let {X(t), t [0,)}

Question:

X(t) = A + Bt, for all t = [0, ∞).

Step by Step Answer:

First note that Similarly xt EXt EAEBt 1t for all t 0 uYtEYt EA ECt 1t ...View the full answer

Introduction To Probability Statistics And Random Processes

Students also viewed these Business questions