Let X 1 , X 2 , X 3 , be independent random variables, where X

Question:

Let X₁, X₂, X₃, ⋯ be independent random variables, where X_n ∼ Bernoulli (1 n) for n = 2, 3,⋯. The goal here is to check whether X_n ^a.s.→ 0.

1. Check that

2. Show that the sequence X₁, X₂, . . . does not converge to 0 almost surely using Theorem 7.6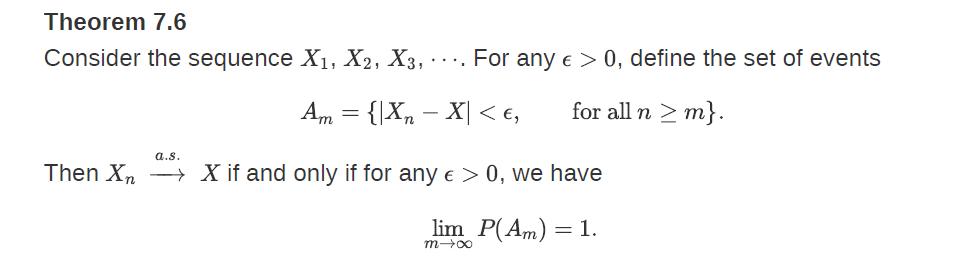 Theorem 7.6 Consider the sequence X, X2, X3, .... For any e > 0, define the set of events Am = {Xn X < for

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Answer rating: 66% (6 reviews)

1 We first note that for 0 1 we have 2 To use Theorem 76 we define Note that for 0 1 we hav...View the full answer

Answered By

Aysha Ali

my name is ayesha ali. i have done my matriculation in science topics with a+ . then i got admission in the field of computer science and technology in punjab college, lahore. i have passed my final examination of college with a+ also. after that, i got admission in the biggest university of pakistan which is university of the punjab. i am studying business and information technology in my university. i always stand first in my class. i am very brilliant client. my experts always appreciate my work. my projects are very popular in my university because i always complete my work with extreme devotion. i have a great knowledge about all major science topics. science topics always remain my favorite topics. i am also a home expert. i teach many clients at my home ranging from pre-school level to university level. my clients always show excellent result. i am expert in writing essays, reports, speeches, researches and all type of projects. i also have a vast knowledge about business, marketing, cost accounting and finance. i am also expert in making presentations on powerpoint and microsoft word. if you need any sort of help in any topic, please dont hesitate to consult with me. i will provide you the best work at a very reasonable price. i am quality oriented and i have 5 year experience in the following field. matriculation in science topics; inter in computer science; bachelors in business and information technology _embed src=http://www.clocklink.com/clocks/0018-orange.swf?timezone=usa_albany& width=200 height=200 wmode=transparent type=application/x-shockwave-flash_

4.40+ 11+ Reviews 14+ Question Solved

Related Book For book-img-for-question

Introduction To Probability Statistics And Random Processes

ISBN: 9780990637202

1st Edition

Authors: Hossein Pishro-Nik

See More Books

Question Posted: Nov 01, 2023 01:39 PM

See More Questions

Let X 1 , X 2 , X 3 , be independent random variables, where X

Question:

∞ Σx=₁ P(|X₂| > €) = ∞. n=1

Step by Step Answer:

1 We first note that for 0 1 we have 2 To use Theorem 76 we define Note that for 0 1 we hav...View the full answer

Introduction To Probability Statistics And Random Processes

Students also viewed these Business questions