Let X have a distribution on the nonnegative integers {0, 1, 2,…} such that (5.18)

holds.

(i) Assuming that E(X) exists, show that

(ii) If we assume that E[X(X − 1)] exists, then it is given by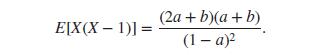

From this, deduce an expression for the variance of X in terms of a and b.
(iii) Combine the results of the first two parts with those of the previous exercise to reestablish the mean and the variance of the Poisson and the negative binomial distributions.
(Hint: Use the identities xf (x) = a(x − 1)f (x − 1) + (a + b)f (x − 1)
and x(x − 1)f (x) = a(x − 1)(x − 2)f (x − 1) + (2a + b)(x − 1)f (x − 1)
and sum the two parts of the first identity for x = 1, 2,…, and of the second identity for x = 2, 3,…)

Transcribed Image Text:

E(X)= = a+b 1- a