Consider a quantum system with just three linearly independent states. Suppose the Hamiltonian, in matrix form, is

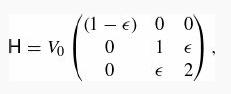where V₀ is a constant, and ϵ is some small number (∈ << 1).
(a) Write down the eigenvectors and eigenvalues of the unperturbed Hamiltonian (∈ = 0).

(b) Solve for the exact eigenvalues of H. Expand each of them as a power series in ϵ, up to second order.
(c) Use first- and second-order non-degenerate perturbation theory to find the approximate eigenvalue for the state that grows out of the nondegenerate eigenvector of H⁰. Compare the exact result, from (b).
(d) Use degenerate perturbation theory to find the first-order correction to the two initially degenerate eigenvalues. Compare the exact results.

Transcribed Image Text:

H = Vo (1 - €) 0 0 1 0 € 0 € 2,