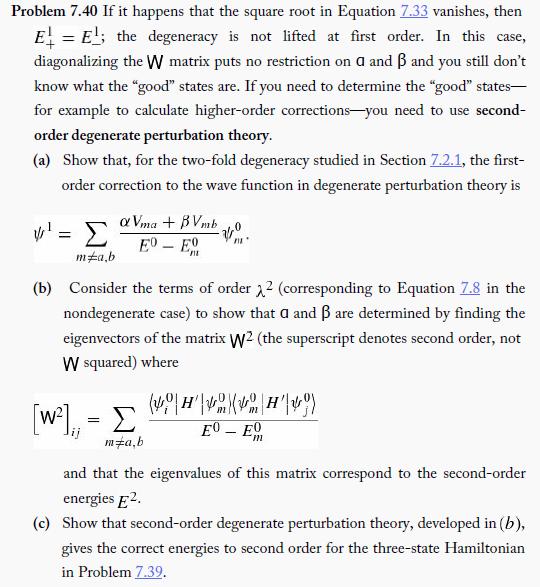
Consider a three-level system with the unperturbed Hamiltonian

(∈_a > ∈_c) and the perturbation

Since the (2x2) matrix W is diagonal (and in fact identically 0) in the basis of states (1,0,0) and (0,1,0), you might assume they are the good states, but they’re not. To see this:
(a) Obtain the exact eigenvalues for the perturbed Hamiltonian H = H⁰ + H'.
(b) Expand your results from part (a) as a power series in |V| up to second order.
(c) What do you obtain by applying nondegenerate perturbation theory to find the energies of all three states (up to second order)? This would work if the assumption about the good states above were correct.

Moral: If any of the eigenvalues of W are equal, the states that diagonalize W are not unique, and diagonalizing W does not determine the “good” states. When this happens (and it’s not uncommon), you need to use second-order degenerate perturbation theory (see Problem 7.40).

= Ea 0 0 0 0 Ea 0 0 Ec (7.111)