Question: Consider the free particle in one dimension: H = p 2 /2m. This Hamiltonian has both translational symmetry and inversion symmetry. (a) Show that translations

Consider the free particle in one dimension: Ĥ = p̂²/2m. This Hamiltonian has both translational symmetry and inversion symmetry.
(a) Show that translations and inversion don’t commute.
(b) Because of the translational symmetry we know that the eigenstates of Ĥ can be chosen to be simultaneous eigenstates of momentum, namely fp (x) (Equation 3.32). Show that the parity operator turns fp(x) into f-p (x); these two states must therefore have the same energy.
(c) Alternatively, because of the inversion symmetry we know that the eigenstates of Ĥ can be chosen to be simultaneous eigenstates of parity, namely

1 h COS px (P) a 1 dsin (P). and

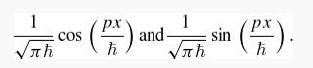
Show that the translation operator mixes these two states together; they therefore must be degenerate.

1 h COS px (P) a 1 dsin (P). and

Step by Step Solution

★★★★★

3.45 Rating (168 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

a Consider operating with the commutator on a test function fx ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Introduction To Quantum Mechanics Questions!

510. Definitions for a multicomponent flash are as follows: x, yi liquid and vapor mole fractions, respectively = feed mole fraction Zj K =y/x-equilibrium constant L,V, F liquid, vapor, and feed...

Consider a particle in one dimension whose Hamiltonian is given by l*(E. - E.) = 2m " alt="Consider a particle in one dimension whose Hamiltonian is given" class="fr-fic fr-dii"> By calculating [[H,...

Are the total energy eigenfunctions for the free particle in one dimension, + (x) A + e +i(2mE/h2)x and (x) A e i(2mE/h2)x eigenfunctions of the one-dimensional linear momentum operator? If so,...

Find (p,t) for the free particle in terms of the function (k) introduced in Equation 2.101. Show that for the free particle | (p,t)| 2 is independent of time. The time independence of | (p,t)| 2...

Consider a free particle in a plane. Using Cartesian coordinates, it is trivial to show that the particle moves in a straight line. The task of this problem is to demonstrate this result in a much...

Explain step by step 3. Consider a particle in a three-dimensional cubic box. a. Calculate the energy for this box, using the Hamiltonian operator, when the particle is in the energy state described...

Consider a free particle in three dimensions; its dynamics is determined by the Hamiltonian H = where P2 = P2 + P2 + P2 and u denotes the mass of the particle. We can define two sets of compatible...

7.1 Let's first consider a free particle, whose wavefunction can be expressed as \begin{equation*} \psi(x, t) = \int_{-\infty}^{\infty} \frac{d p}{\sqrt{2 \pi \hbar}} g(p) e^{-i \frac{E_{p} t - p...

5. 2-dimentional box Consider a free particle contained in 2-dimensional impenetrable square as below: 0 a X b y (a) Set up a time-independent Schrodinger equation for the particle (b) What is the...

Consider a free particle moving in one dimension whose probability of moving in the positive x direction is four times that for moving in the negative x direction. Give as much information as you can...

What is the current price of a share of stock when the last dividend was $3.00, the growth rate is 6 percent, and the investor's required rate of return is 12 percent? $25.00 $26.50 $50.00 $53.00

Write down the various power bases that you think your parents or immediate family members hold over you. In what ways do you yield to the power they exert? Or, do you resist the power they try to...

Ben, who uses the cash method of accounting, lives in a state that imposes an income tax ( including withholding from wages ) . On April 1 4 , 2 0 2 4 , he flles his state return for 2 0 2 3 , paying...

The motor of a crane uses power P to lift a steel beam. By what factor must the motors power increase to lift the beam twice as high in half the time?

A flexible L-shaped raft shown in Figure P8.9 applies a uniform pressure of 60 kN/m 2 to the underlying ground. Find the vertical stress increase at 4 m below A, B, and C. Figure P8.9 - 6 m - 8 m 4...

A square flexible foundation of width B applies a uniform pressure q o to the underlying ground. Determine the vertical stress increase at a depth of B/2 below the center using: a. beneath the...

A square foundation, 1.5 m wide, carries a net column load of 500 kN as shown in Figure P8.11. Determine the average stress increase beneath the center of the foundation in the clay layer: a. Using...

Your company has $ 2 3 0 million to spend in Year 1 on capital investment projects. The Year 1 required spending, mean NPV , and sigma of the NPV for each project are shown in the spreadsheet. Also...

If the nominal rate of return on a risky security is 9 percent, and the risk-free rate is 4.9 percent, what is the risk premium? 7.48%4.10%3.91%2.24% QUESTION 9 The rate of interest on a financial...

A student loan is offered under the following conditions: credit of 3 0 , 0 0 0 euros at 3 % over a period of 6 years contracted on June 1 , 2 0 2 0 ; no repayment or interest payments for 2 years;...