In Problem 11.1 you showed that the solution to (where k(t) is a function of t) is
Question:
In Problem 11.1 you showed that the solution to
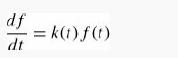
(where k(t) is a function of t) is

This suggests that the solution to the Schrödinger equation (11.1) might be
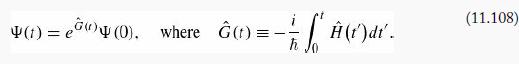
It doesn’t work, because Ĥ(t) is an operator, not a function, and Ĥ(t1) does not (in general) commute with Ĥ(t2).
(a) Try calculating iћ∂Ψ/∂t, using Equation 11.108.
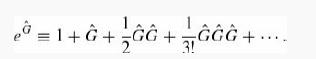
Show that if [Ĝ,Ĥ] = 0 then Ψ satisfies the Schrödinger equation
(b) Check that the correct solution in the general case ([Ĝ,Ĥ] ≠ 0) is
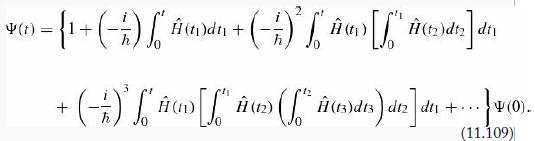
UGLY! Notice that the operators in each term are “time-ordered,” in the sense that the latest Ĥ appears at the far left, followed by the next latest, and so on (t ≥ t1 ≥ t2 ≥ t3 ...). Dyson introduced the time-ordered product of two operators:
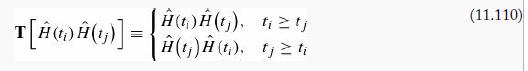
or, more generally,
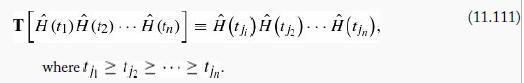
(c) Show that
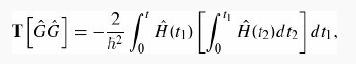
and generalize to higher powers of Ĝ. In place of Ĝn, in equation 11.108, we really want T [Ĝn]:

This is Dyson’s formula; it’s a compact way of writing Equation 11.109, the formal solution to Schrödinger’s equation. Dyson’s formula plays a fundamental role in quantum field theory.
![]()
Step by Step Answer:

Introduction To Quantum Mechanics
ISBN: 9781107189638
3rd Edition
Authors: David J. Griffiths, Darrell F. Schroeter





