Let (Pi(z)) be the probability generating function of the limiting distribution (pi) for the (M / G

Question:

Let $\Pi(z)$ be the probability generating function of the limiting distribution $\pi$ for the $M / G / 1$ queue, and let $Q(z)$ be the probability generating function of the distribution $\left(q_{n}\right)$ in (11). Multiply both sides of (13) by $z^{i}$ and sum from $i=0$ to $\infty$ to obtain the relation

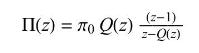
image text in transcribed