An econometrician wishes to study the properties of an estimator using simulated data. Suppose the sample size \(N\) is set to be 100 . The intercept and slope parameters are 100 , and 10 , respectively. The one explanatory variable, \(x\), has a normal distribution with mean 10 and standard deviation 10 . A standard normal random variable, \(z\), independent of \(x\), is created. The data generating process is \(y_{i}=\beta_{1}+\beta_{2} x_{i}+e_{i}\), where

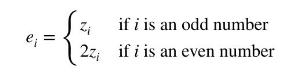
a. The OLS estimator is not the best linear unbiased estimator using the 100 data pairs \(\left(y_{i}, x_{i}\right)\). True or false? Explain.

b. If we divide \(y\) and \(x\) for the even number observations by \(\sqrt{2}\), leaving the odd number observations alone, and then run a least squares regression, the resulting estimator is BLUE. True or false? Explain.

c. Suppose you were assigned the task of showing that the heteroskedasticity in the data was "statistically significant." Using the 100 data pairs \(\left(y_{i}, x_{i}\right)\), how exactly would you do it?

Transcribed Image Text:

= Zi 2z; if i is an odd number if i is an even number