Consider a translog production function where output is measured as firm sales and there are three inputs: capital, labor, and materials. This function can be written as

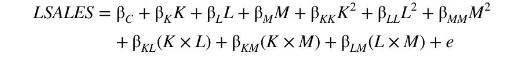
where LSALES is the log of sales, and \(K, L\), and \(M\) are the logs of capital, labor and materials, respectively. The translog function is often known as a flexible functional form, intended to approximate a variety of possible functional forms. There are two hypotheses that are likely to be of interest:

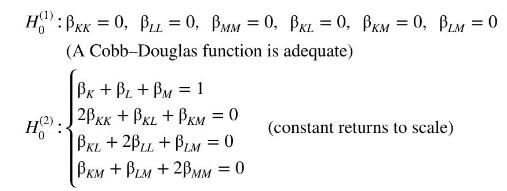
The data file chemical_small contains observations on 1200 firms in China's chemical industry, taken in the year 2006. It is a subset of the data used by Baltagi, Egger, and Kesina \({ }^{19}\).

a. Use these data to estimate the translog production function. Are all the coefficient estimates significant at a \(5 \%\) level of significance?

b. Test \(H_{0}^{(1)}\) at a \(5 \%\) level of significance.

c. Test \(H_{0}^{(2)}\) at a \(5 \%\) level of significance. What would be the test outcome if you used a \(1 \%\) level of significance?

d. Does RESET suggest the translog function is adequate?

e. Estimate the model with the restrictions implied by constant returns to scale \(\left(H_{0}^{(2)}\right)\) imposed. Obtain estimates and standard errors for all 10 coefficients.

f. Compare the estimates and standard errors from parts (a) and (e).

g. Does RESET suggest the restricted model is adequate?

Transcribed Image Text:

LSALES = c+xK+L +MM+BKK K + BLLL + MMM +BKL (KXL) + BKM(KM) + BLM (LXM)+e