Consider the following five observations. You are to do all the parts of this exercise using only a calculator.

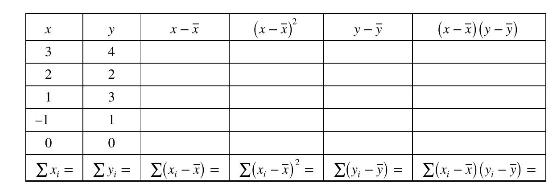
a. Complete the entries in the table. Put the sums in the last row. What are the sample means \(\bar{x}\) and \(\bar{y}\) ?

b. Calculate \(b_{1}\) and \(b_{2}\) using (2.7) and (2.8) and state their interpretation.

c. Compute \(\sum_{i=1}^{5} x_{i}^{2}, \sum_{i=1}^{5} x_{i} y_{i}\). Using these numerical values, show that \(\sum\left(x_{i}-\bar{x}\right)^{2}=\sum x_{i}^{2}-N \bar{x}^{2}\) and \(\sum\left(x_{i}-\bar{x}\right)\left(y_{i}-\bar{y}\right)=\sum x_{i} y_{i}-N \bar{x} \bar{y}\).

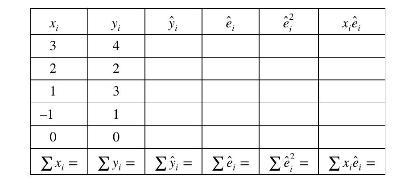
d. Use the least squares estimates from part (b) to compute the fitted values of \(y\), and complete the remainder of the table below. Put the sums in the last row.
Calculate the sample variance of \(y, s_{y}^{2}=\sum_{i=1}^{N}\left(y_{i}-\bar{y}\right)^{2} /(N-1)\), the sample variance of \(x\), \(s_{x}^{2}=\sum_{i=1}^{N}\left(x_{i}-\bar{x}\right)^{2} /(N-1)\), the sample covariance between \(x\) and \(y, s_{x y}=\sum_{i=1}^{N}\left(y_{i}-\bar{y}\right)\left(x_{i}-\bar{x}\right) /\) \((N-1)\), the sample correlation between \(x\) and \(y, r_{x y}=s_{x y} /\left(s_{x} s_{y}\right)\) and the coefficient of variation of \(x, C V_{x}=100\left(s_{x} / \bar{x}\right)\). What is the median, 50th percentile, of \(x\) ?

e. On graph paper, plot the data points and sketch the fitted regression line \(\hat{y}_{i}=b_{1}+b_{2} x_{i}\).

f. On the sketch in part (e), locate the point of the means \((\bar{x}, \bar{y})\). Does your fitted line pass through that point? If not, go back to the drawing board, literally.

g. Show that for these numerical values \(\bar{y}=b_{1}+b_{2} \bar{x}\).

h. Show that for these numerical values \(\overline{\hat{y}}=\bar{y}\), where \(\overline{\hat{y}}=\sum \hat{y}_{i} / N\).

i. Compute \(\hat{\sigma}^{2}\).

j. Compute \(\widehat{\operatorname{var}}\left(b_{2} \mid \mathbf{x}\right)\) and \(\operatorname{se}\left(b_{2}\right)\).

Transcribed Image Text:

x y (x-5) y-y (x-x)(y-y) rr 3 4 2 2 1 3 -I 0 1 0 2 = = (x -1) = (-1) = ( - ) = (-) (-1) =