Consider the regression model (y_{i t}=beta_{1}+beta_{2} x_{2 i t}+alpha_{1} w_{1 i}+u_{i}+e_{i t}, i=1, ldots, N, t=1, ldots,
Question:
Consider the regression model \(y_{i t}=\beta_{1}+\beta_{2} x_{2 i t}+\alpha_{1} w_{1 i}+u_{i}+e_{i t}, i=1, \ldots, N, t=1, \ldots, T\), where \(x_{2 i t}\) and \(w_{1 i}\) are explanatory variables. The time-averaged model is given in equation (15.13), \(\bar{y}_{i \bullet}=\beta_{1}+\beta_{2} \bar{x}_{2 i \bullet}+\alpha_{1} w_{1 i}+\bar{v}_{i \bullet}\) where \(\bar{v}_{i \bullet}=u_{i}+\bar{e}_{i \bullet}\). The OLS estimator of the parameters in (15.13) is called the between estimator, because it uses variation between, or among, individuals to estimate the regression parameters.
a. Under assumptions RE1-RE5, derive the variance of the random error \(\bar{v}_{i}=u_{i}+\bar{e}_{i .}\).
b. Under assumptions RE1-RE5, find the covariance between \(\bar{v}_{i}\), and \(\bar{v}_{j}\), where \(i eq j\).
c. Under assumptions RE1-RE5, the between estimator is unbiased. Is this true or false? Explain the basis of your answer.
d. If assumptions RE1-RE5 hold except for RE2, part (ii), then the between estimator is biased and inconsistent. Is this true or false? Explain the basis of your answer.
Data From Equation 15.13:-
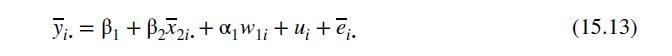
Step by Step Answer:

Principles Of Econometrics
ISBN: 9781118452271
5th Edition
Authors: R Carter Hill, William E Griffiths, Guay C Lim





