For positive random variables X and Y, suppose the expected value of Y given X is E(Y|X) = θX. The unknown parameter u shows how the expected value of Y changes with X.

(i) Define the random variable Z = Y/X. Show that E(Z) = θ.

(ii) Use part (i) to prove that the estimator W₁ = n^–1Σⁿ_i=1 (Y_i/X_i) is unbiased for θ, where {(X_i, Y_i): i = 1, 2, . . . , n6 is a random sample.

(iii) Explain why the estimator W₂ = Y̅/X̅, where the overbars denote sample averages, is not the same as W₁. Nevertheless, show that W₂ is also unbiased for θ.

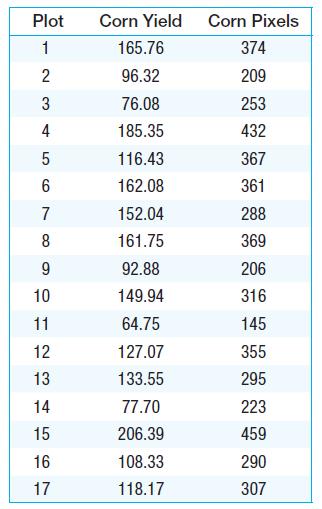
(iv) The following table contains data on corn yields for several counties in Iowa. The USDA predicts the number of hectares of corn in each county based on satellite photos. Researchers count the number of “pixels” of corn in the satellite picture (as opposed to, for example, the number of pixels of soybeans or of uncultivated land) and use these to predict the actual number of hectares. To develop a prediction equation to be used for counties in general, the USDA surveyed farmers in selected counties to obtain corn yields in hectares. Let Y_i = corn yield in county i and let X_i = number of corn pixels in the satellite picture for county i. There are n = 17 observations for eight counties. Use this sample to compute the estimates of u devised in parts (ii) and (iii). Are the estimates similar?

Transcribed Image Text:

Plot Corn Yield Corn Pixels 1 165.76 374 96.32 209 76.08 253 4 185.35 432 116.43 367 162.08 361 7 152.04 288 8 161.75 369 92.88 206 10 149.94 316 11 64.75 145 12 127.07 355 13 133.55 295 14 77.70 223 15 206.39 459 16 108.33 290 17 118.17 307 2. 3.